如果你也在 怎样代写抽象代数abstract algebra这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。
抽象代数是代数的一组高级课题,涉及抽象代数结构而不是通常的数系。这些结构中最重要的是群、环和场。
statistics-lab™ 为您的留学生涯保驾护航 在代写抽象代数abstract algebra方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写抽象代数abstract algebra代写方面经验极为丰富,各种代写抽象代数abstract algebra相关的作业也就用不着说。
我们提供的抽象代数abstract algebra及其相关学科的代写,服务范围广, 其中包括但不限于:
- Statistical Inference 统计推断
- Statistical Computing 统计计算
- Advanced Probability Theory 高等概率论
- Advanced Mathematical Statistics 高等数理统计学
- (Generalized) Linear Models 广义线性模型
- Statistical Machine Learning 统计机器学习
- Longitudinal Data Analysis 纵向数据分析
- Foundations of Data Science 数据科学基础
数学代写|抽象代数作业代写abstract algebra代考|Noncommutative ring theory
Algebra textbooks usually give the definition of a ring first and follow it with examples. Of course the examples came first, and the abstract definition later-much later. So we begin with examples.
Among the most important examples of rings are the integers, polynomials, and matrices. “Simple” extensions of these examples are at the roots of ring theory. Specifically, we have in mind the following three examples:
(a) The integers $Z$ can be thought of as the appropriate subdomain of the field $Q$ of rationals in which to do number theory. (The rationals themselves are unsuitable for that purpose: every rational is divisible by every other (nonzero) rational.) Take a simple extension field $Q(\alpha)$ of the rationals, where $\alpha$ is an algebraic number, that is, a root of a polynomial with integer coefficients. $Q(\alpha)$ is called an algebraic number field; it consists of polynomials in $\alpha$ with rational coefficients. For example, $Q(\sqrt{3})={a+b \sqrt{3}: a, b \in Q}$.
The appropriate subdomain of $Q(\alpha)$ in which to do number theory -the “integers” of $Q(\alpha)$-consists of those elements that are roots of monic polynomials with integer coefficients, polynomials $p(x)$ in which the coefficient of the highest power of $x$ is 1 . For example, the integers of $Q(\sqrt{3})$ are ${a+b \sqrt{3}: a, b \in Z}$ (this is not obvious). This is our first example.
(b) The polynomial rings $\mathbf{R}[x]$ and $\mathbf{R}[x, y]$ in one and in two variables, respectively, share important properties but also differ in significant ways ( $\mathbf{R}$ denotes the real numbers). In particular, while the roots of a polynomial in one variable constitute a discrete set of real numbers, the roots of a polynomial in two variables constitute a curve in the plane-a so-called algebraic curve. Our second example, then, is the ring of polynomials in two (or more) variables.
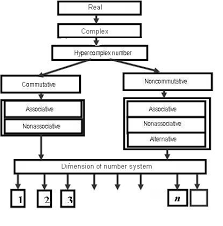
(c) Square $m \times m$ matrices (for example, over the reals) can be viewed as $m^{2}$ tuples of real numbers with coordinate-wise addition and appropriate multiplication obeying the axioms of a ring. Our third example consists, more generally, of $n$-tuples $\mathbf{R}^{n}$ of real numbers with coordinate-wise addition and appropriate multiplication, so that the resulting system is a (not necessarily commutative) ring. Such systems, often extensions of the complex numbers, were called in the nineteenth and early twentieth centuries hypercomplex number systems.
In what contexts did these examples arise? What was their importance? The answers will lead us to the genesis of ring theory.
Rings fall into two broad categories: commutative and noncommutative. The abstract theories of these two categories came from distinct sources and developed in different directions. Commutative ring theory originated in algebraic number theory, algebraic geometry, and invariant theory. Central to the development of these subjects were the rings of integers in algebraic number fields and algebraic function fields, and the rings of polynomials in two or more variables.
Noncommutative ring theory began with attempts to extend the complex numbers to various hypercomplex number systems. The genesis of the theories of commutative and noncommutative rings dates back to the early nineteenth century, while their maturity was achieved only in the third decade of the twentieth century.
The following is a diagrammatic sketch of the above remarks.
数学代写|抽象代数作业代写abstract algebra代考|Examples of Hypercomplex Number Systems
Hamilton’s invention of the quaternions was conceptually groundbreaking-_”a revolution in arithmetic which is entirely similar to the one which Lobachevsky effected
in geometry,” according to Poincaré. Indeed, both achievements were radical violations of prevailing conceptions. Like all revolutions, however, the invention of the quaternions was initially received with less than universal approbation: “I have not yet any clear view as to the extent to which we are at liberty arbitrarily to create imaginaries, and to endow them with supernatural properties,” declared Hamilton’s mathematician friend John Graves.
Most mathematicians, however, including Graves, soon came around to Hamilton’s point of view. The quaternions acted as a catalyst for the exploration of diverse “number systems,” with properties which departed in various ways from those of the real and complex numbers. Among the examples of such hypercomplex number systems are the following:
(i) Octonions
These are 8-tuples of reals which contain the quaternions and form a division algebra in which multiplication is nonassociative. They were introduced in 1844 by Cayley and independently by the very John Graves who questioned Hamilton’s “imaginaries.”
(ii) Exterior algebras
These are $n$-tuples of reals, added componentwise and multiplied via the “exterior product.” They were introduced by Grassmann in 1844 as part of a brilliant attempt to construct a vector algehra in $n$-dimensional space. Grassmann’s style was far from simple and his approach was ahead of its time.
(iii) Group algebras
In 1854 Cayley published a paper on (finite) abstract groups, at the end of which he gave a definition of a group algebra (over the real or complex numbers). He called it a system of “complex quantities” and observed that it is analogous to Hamilton’s quaternions-it is associative, noncommutative, but in general not a division algebra.
(iv) Matrices
In two papers of 1855 and 1858 Cayley introduced square matrices. He noted that they can be treated as “single quantities,” added and multiplied like “ordinary algebraic quantities,” but that “as regards their multiplication, there is the peculiarity that matrices are not in general convertible [commutative].” See Chapter 5.1.3.
数学代写|抽象代数作业代写abstract algebra代考|Classification
Over a thirty-year period (c. $1840-1870$ ) a stock of examples of noncommutative number systems had been established. One could now begin to construct a theory. The general concept of a hypercomplex number system (in current terminology, a finite-dimensional algebra) emerged, and work began on classifying certain types
of these structures. We focus on three such developments, dealing with associative algebras. Note that such algebras are, of course, rings.
(i) Low-dimensional algebras
Of fundamental importance here was the work of Benjamin Peirce of Harvard-the first important contribution to algebra in the U.S. We are referring to his groundbreaking paper “Linear Associative Algebra” of 1870. In the last 100 pages of this 150-page paper Peirce classified algebras (i.e., hypercomplex number systems) of dimension $<6$ by giving their multiplication tables. There are, he showed, over 150 such algebras! What is important in this paper, though, is not the classification but the means used to obtain it. For here Peirce introduced concepts, and derived results, which proved fundamental for subsequent developments. Among these conceptual advances were:
(a) An “abstract” definition of a finite-dimensional algebra. Peirce defined such an algebra-he called it a “linear associative algebra”-as the totality of formal expressions of the form $\sum_{i=1}^{n} a_{i} e_{i}$, where the $e_{i}$ are “basis elements.” Addition was defined componentwise and multiplication by means of “structural constants” $c_{i j k}$, namely $e_{i} e_{j}=\sum_{k=1}^{n} c_{i j k} e_{k}$. Associativity under multiplication and distributivity were postulated, but not commutativity. This is probably the earliest explicit definition of an associative algebra.
(b) The use of complex coefficients. Peirce took the coefficients $a_{i}$ in the expressions $\sum a_{i} e_{i}$ to be complex numbers. This conscious broadening of the field of coefficients from $\mathbf{R}$ to $\mathbf{C}$ was an important conceptual advance on the road to coefficients taken from an arbitrary field.
(c) Relaxation of the requirement that an algebra have an identity. This, too, was a departure from past practice and gave an indication of Peirce’s general, abstract approach.
抽象代数代写
数学代写|抽象代数作业代写abstract algebra代考|Noncommutative ring theory
代数教科书通常先给出环的定义,然后再举例子。当然,例子是最先出现的,抽象的定义在后面——要晚得多。所以我们从例子开始。
环中最重要的例子是整数、多项式和矩阵。这些例子的“简单”扩展是环理论的根源。具体来说,我们想到了以下三个例子:
(a) 整数从可以认为是该领域的适当子域问做数论的有理数。(有理数本身不适合这个目的:每个有理数都可以被其他(非零)有理数整除。) 取一个简单的外延域问(一种)的有理数,其中一种是一个代数数,即具有整数系数的多项式的根。问(一种)称为代数数域;它由多项式组成一种有理系数。例如,问(3)=一个+b3:一个,b∈问.
适当的子域问(一种)在其中做数论 – 的“整数”问(一种)-由那些是具有整数系数的一元多项式的根的元素组成,多项式p(X)其中的最高功率系数X是 1 。例如,整数问(3)是一个+b3:一个,b∈从(这并不明显)。这是我们的第一个例子。
(b) 多项式环R[X]和R[X,是]在一个和两个变量中,分别具有重要的属性,但也存在显着差异(R表示实数)。特别是,多项式在一个变量中的根构成一组离散的实数,而多项式在两个变量中的根构成平面中的一条曲线——所谓的代数曲线。那么,我们的第二个例子是两个(或更多)变量的多项式环。
(c) 广场米×米矩阵(例如,在实数上)可以被视为米2符合环公理的坐标加法和适当乘法的实数元组。我们的第三个例子更一般地包括n-元组Rn具有坐标加法和适当乘法的实数,因此得到的系统是(不一定是可交换的)环。这种系统,通常是复数的扩展,在 19 世纪和 20 世纪初被称为超复数系统。
这些例子是在什么背景下出现的?它们的重要性是什么?答案将引导我们了解环理论的起源。
环分为两大类:可交换的和不可交换的。这两个范畴的抽象理论来源不同,发展方向不同。交换环论起源于代数数论、代数几何和不变量论。这些学科发展的核心是代数数域和代数函数域中的整数环,以及两个或多个变量中的多项式环。
非交换环理论开始于尝试将复数扩展到各种超复数系统。交换环和非交换环理论的起源可以追溯到 19 世纪初,而它们的成熟是在 20 世纪 30 年代才实现的。
以下是上述评论的示意图。
数学代写|抽象代数作业代写abstract algebra代考|Examples of Hypercomplex Number Systems
汉密尔顿对四元数的发明在概念上是开创性的——“一场算术革命,与罗巴切夫斯基实现的革命完全相似
几何学,”根据庞加莱的说法。事实上,这两项成就都彻底违反了主流观念。然而,像所有的革命一样,四元数的发明最初并没有得到普遍认可:“我还没有清楚地看到我们在多大程度上可以任意创造想象,并赋予它们超自然的特性,”汉密尔顿的数学家朋友约翰格雷夫斯宣称。
然而,包括格雷夫斯在内的大多数数学家很快就接受了汉密尔顿的观点。四元数充当了探索各种“数系”的催化剂,其性质以各种方式偏离实数和复数的性质。此类超复数系统的示例如下:
(i) Octonions
这些是实数的 8 元组,包含四元数并形成除法代数,其中乘法是非关联的。它们是由 Cayley 于 1844 年引入的,并且是由质疑汉密尔顿的“想象”的 John Graves 独立提出的。
(ii) 外代数
这些是n-实数元组,按分量相加并通过“外积”相乘。它们是格拉斯曼在 1844 年引入的,作为在n维空间。格拉斯曼的风格远非简单,他的方法领先于时代。
(iii) 群代数
1854 年,Cayley 发表了一篇关于(有限)抽象群的论文,最后他给出了群代数(在实数或复数上)的定义。他称其为“复量”系统,并观察到它类似于汉密尔顿的四元数——它是结合的、不可交换的,但通常不是除法代数。
(iv) 矩阵
在 1855 年和 1858 年的两篇论文中,凯莱介绍了方阵。他指出,它们可以被视为“单个量”,可以像“普通代数量”一样相加和相乘,但是“关于它们的乘法,有一个特点,即矩阵通常不可转换 [commutative]。” 请参见第 5.1.3 章。
数学代写|抽象代数作业代写abstract algebra代考|Classification
超过三十年(c.1840−1870) 已经建立了一组非交换数系统的例子。现在可以开始构建一种理论了。超复数系统的一般概念(在当前术语中,有限维代数)出现了,并且开始对某些类型进行分类
这些结构中。我们专注于三个这样的发展,处理关联代数。请注意,这样的代数当然是环。
(i) 低维代数
这里最重要的是哈佛 Benjamin Peirce 的工作——美国对代数的第一个重要贡献 我们指的是他 1870 年的开创性论文“线性关联代数”。在最后 100 页这篇 150 页的论文 Peirce 对维度的代数(即超复数系统)进行了分类<6通过给出他们的乘法表。他展示了超过 150 个这样的代数!然而,本文重要的不是分类,而是用于获得分类的方法。在这里,Peirce 引入了概念,并得出了结果,这些结果证明是后续发展的基础。这些概念上的进步包括:
(a) 有限维代数的“抽象”定义。Peirce 将这样一个代数——他称之为“线性结合代数”——定义为以下形式的形式表达式的总和∑一世=1n一个一世和一世, 其中和一世是“基本要素”。加法是通过“结构常数”定义的组件和乘法C一世jķ,即和一世和j=∑ķ=1nC一世jķ和ķ. 假设乘法和分配下的关联性,但不是交换性。这可能是关联代数最早的明确定义。
(b) 复系数的使用。皮尔斯取系数一个一世在表达式中∑一个一世和一世是复数。这种有意识地扩大系数领域R至C是从任意领域获取系数的重要概念进步。
(c) 放宽代数有恒等式的要求。这也与过去的做法背道而驰,并表明了皮尔士的一般抽象方法。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。
金融工程代写
金融工程是使用数学技术来解决金融问题。金融工程使用计算机科学、统计学、经济学和应用数学领域的工具和知识来解决当前的金融问题,以及设计新的和创新的金融产品。
非参数统计代写
非参数统计指的是一种统计方法,其中不假设数据来自于由少数参数决定的规定模型;这种模型的例子包括正态分布模型和线性回归模型。
广义线性模型代考
广义线性模型(GLM)归属统计学领域,是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。
术语 广义线性模型(GLM)通常是指给定连续和/或分类预测因素的连续响应变量的常规线性回归模型。它包括多元线性回归,以及方差分析和方差分析(仅含固定效应)。
有限元方法代写
有限元方法(FEM)是一种流行的方法,用于数值解决工程和数学建模中出现的微分方程。典型的问题领域包括结构分析、传热、流体流动、质量运输和电磁势等传统领域。
有限元是一种通用的数值方法,用于解决两个或三个空间变量的偏微分方程(即一些边界值问题)。为了解决一个问题,有限元将一个大系统细分为更小、更简单的部分,称为有限元。这是通过在空间维度上的特定空间离散化来实现的,它是通过构建对象的网格来实现的:用于求解的数值域,它有有限数量的点。边界值问题的有限元方法表述最终导致一个代数方程组。该方法在域上对未知函数进行逼近。[1] 然后将模拟这些有限元的简单方程组合成一个更大的方程系统,以模拟整个问题。然后,有限元通过变化微积分使相关的误差函数最小化来逼近一个解决方案。
tatistics-lab作为专业的留学生服务机构,多年来已为美国、英国、加拿大、澳洲等留学热门地的学生提供专业的学术服务,包括但不限于Essay代写,Assignment代写,Dissertation代写,Report代写,小组作业代写,Proposal代写,Paper代写,Presentation代写,计算机作业代写,论文修改和润色,网课代做,exam代考等等。写作范围涵盖高中,本科,研究生等海外留学全阶段,辐射金融,经济学,会计学,审计学,管理学等全球99%专业科目。写作团队既有专业英语母语作者,也有海外名校硕博留学生,每位写作老师都拥有过硬的语言能力,专业的学科背景和学术写作经验。我们承诺100%原创,100%专业,100%准时,100%满意。
随机分析代写
随机微积分是数学的一个分支,对随机过程进行操作。它允许为随机过程的积分定义一个关于随机过程的一致的积分理论。这个领域是由日本数学家伊藤清在第二次世界大战期间创建并开始的。
时间序列分析代写
随机过程,是依赖于参数的一组随机变量的全体,参数通常是时间。 随机变量是随机现象的数量表现,其时间序列是一组按照时间发生先后顺序进行排列的数据点序列。通常一组时间序列的时间间隔为一恒定值(如1秒,5分钟,12小时,7天,1年),因此时间序列可以作为离散时间数据进行分析处理。研究时间序列数据的意义在于现实中,往往需要研究某个事物其随时间发展变化的规律。这就需要通过研究该事物过去发展的历史记录,以得到其自身发展的规律。
回归分析代写
多元回归分析渐进(Multiple Regression Analysis Asymptotics)属于计量经济学领域,主要是一种数学上的统计分析方法,可以分析复杂情况下各影响因素的数学关系,在自然科学、社会和经济学等多个领域内应用广泛。
MATLAB代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。
