标签： Math 417

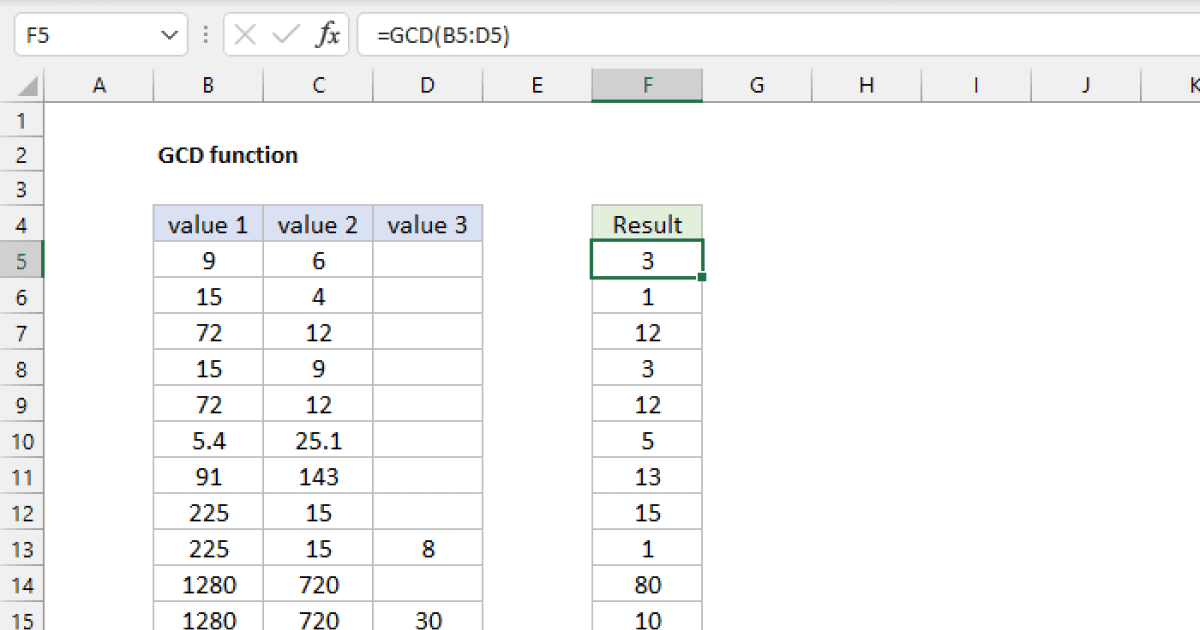
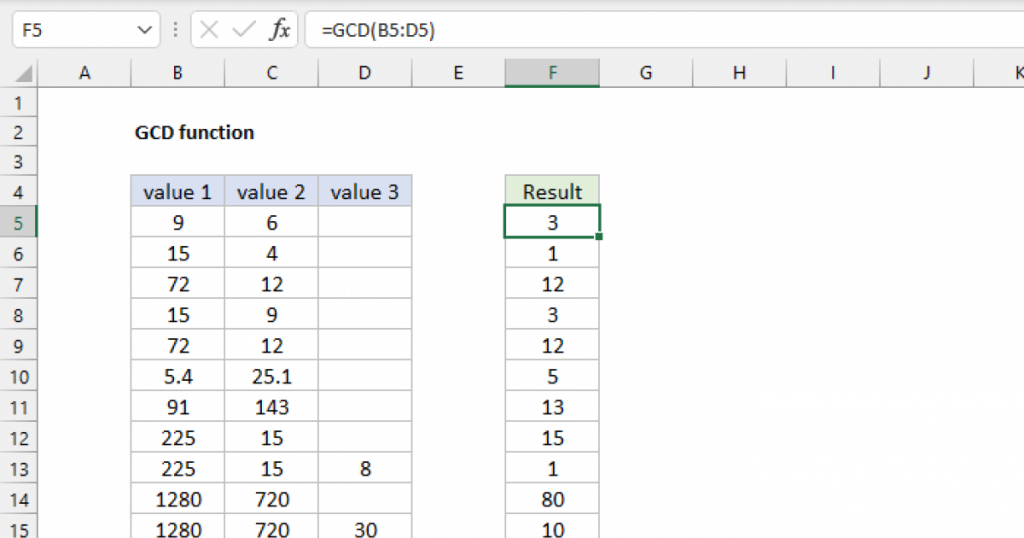
数学代写|现代代数代写Modern Algebra代考|Definition of a Group

Posted on 2023年7月13日2023年7月13日 by statistics-lab

如果你也在怎样代写现代代数Modern Algebra 这个学科遇到相关的难题，请随时右上角联系我们的24/7代写客服。现代代数Modern Algebra有时被称为代数结构或抽象代数，或者仅仅在高等数学的背景下被称为代数。虽然这个名字可能只是暗示了一种新的方式来表示微积分之前的代数，但实际上它比微积分更广泛、更深入。

现代代数Modern Algebra这门学科的思想和方法几乎渗透到现代数学的每一个部分。此外，没有一门学科更适合培养处理抽象概念的能力，即理解和处理问题或学科的基本要素。这包括阅读数学的能力，提出正确的问题，解决问题，运用演绎推理，以及写出正确、切中要害、清晰的数学。

statistics-lab™ 为您的留学生涯保驾护航在代写现代代数Modern Algebra方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写现代代数Modern Algebra代写方面经验极为丰富，各种代写现代代数Modern Algebra相关的作业也就用不着说。

数学代写|现代代数代写Modern Algebra代考|Definition of a Group

The fundamental notions of set, mapping, binary operation, and binary relation were presented in Chapter 1. These notions are essential for the study of an algebraic system. An algebraic structure, or algebraic system, is a nonempty set in which at least one equivalence relation (equality) and one or more binary operations are defined. The simplest structures occur when there is only one binary operation, as is the case with the algebraic system known as a group.
An introduction to the theory of groups is presented in this chapter, and it is appropriate to point out that this is only an introduction. Entire books have been devoted to the theory of groups; the group concept is extremely useful in both pure and applied mathematics.
A group may be defined as follows.
Group
Suppose the binary operation * is defined for elements of the set $G$. Then $G$ is a group with respect to * provided the following four conditions hold:

$G$ is closed under *. That is, $x \in G$ and $y \in G$ imply that $x * y$ is in $G$.
* is associative. For all $x, y, z$ in $G, x *(y * z)=(x * y) * z$.
$G$ has an identity element $e$. There is an $e$ in $G$ such that $x * e=e * x=x$ for all $x \in G$.
$G$ contains inverses. For each $a \in G$, there exists $b \in G$ such that $a * b=b * a=e$.

The phrase “with respect to *” should be noted. For example, the set $\mathbf{Z}$ of all integers is a group with respect to addition but not with respect to multiplication (it has no inverses for elements other than \pm 1 ). Similarly, the set $G={1,-1}$ is a group with respect to multiplication but not with respect to addition. In most instances, however, only one binary operation is under consideration, and we say simply that ” $G$ is a group.” If the binary operation is unspecified, we adopt the multiplicative notation and use the juxtaposition $x y$ to indicate the result of combining $x$ and $y$. Keep in mind, though, that the binary operation is not necessarily multiplication.

数学代写|现代代数代写Modern Algebra代考|Properties of Group Elements

Several consequences of the definition of a group are recorded in Theorem 3.4.
Parts $\mathbf{a}$ and $\mathbf{b}$ of the next theorem are statements about uniqueness, and they can be proved by the standard type of uniqueness proof: Assume that two such quantities exist, and then prove the two to be equal.
Properties of Group Elements
Let $G$ be a group with respect to a binary operation that is written as multiplication.
a. The identity element $e$ in $G$ is unique.
b. For each $x \in G$, the inverse $x^{-1}$ in $G$ is unique.
c. For each $x \in G,\left(x^{-1}\right)^{-1}=x$.
d. Reverse order law. For any $x$ and $y$ in $G,(x y)^{-1}=y^{-1} x^{-1}$.
e. Cancellation laws. If $a, x$, and $y$ are in $G$, then either of the equations $a x=a y$ or $x a=y a$ implies that $x=y$.

Proof We prove parts $\mathbf{b}$ and $\mathbf{d}$ and leave the others as exercises. To prove part $\mathbf{b}$, let $x \in G$, and suppose that each of $y$ and $z$ is an inverse of $x$. That is,
$$
x y=e=y x \text { and } x z=e=z x .
$$
Then
$$
\begin{aligned}
y & =e y & & \text { since } e \text { is an identity } \
& =(z x) y & & \text { since } z x=e \
& =z(x y) & & \text { by associativity } \
& =z(e) & & \text { since } x y=e \
& =z & & \text { since } e \text { is an identity. }
\end{aligned}
$$
Thus $y=z$, and this justifies the notation $x^{-1}$ as the unique inverse of $x$ in $G$.
We shall use part $\mathbf{b}$ in the proof of part $\mathbf{d}$. Specifically, we shall use the fact that the inverse $(x y)^{-1}$ is unique. This means that in order to show that $y^{-1} x^{-1}=(x y)^{-1}$, we only need to verify that $(x y)\left(y^{-1} x^{-1}\right)=e=\left(y^{-1} x^{-1}\right)(x y)$. These calculations are straightforward:
$$
\left(y^{-1} x^{-1}\right)(x y)=y^{-1}\left(x^{-1} x\right) y=y^{-1} e y=y^{-1} y=e
$$
and
$$
(x y)\left(y^{-1} x^{-1}\right)=x\left(y y^{-1}\right) x^{-1}=x e x^{-1}=x x^{-1}=e .
$$

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Definition of a Group

第一章给出了集合、映射、二元运算和二元关系的基本概念。这些概念对于代数系统的研究是必不可少的。代数结构或代数系统是定义了至少一个等价关系(等式)和一个或多个二元操作的非空集合。最简单的结构出现在只有一个二进制运算的时候，就像被称为群的代数系统一样。
在本章中介绍了群的理论，并适当地指出，这只是一个介绍。整本整本的书都致力于群体理论;群的概念在纯数学和应用数学中都非常有用。
组可以定义如下。
集团
假设为集合$G$中的元素定义了二元操作。则$G$是关于的群，只要满足以下四个条件:

$G$在*下关闭。也就是说，$x \在G$中，$y \在G$中意味着$x * y$在G$中。

*是结合律。对于$G中的所有$x, y, z$， x *(y * z)=(x * y) * z$。

$G$有一个单位元$e$。在$G$中有一个$e$使得$x * e=e * x=x$对于G$中的所有$x \。

$G$包含逆。对于G$中的每一个$a \，在G$中存在$b \使得$a * b=b * a=e$。

应该注意短语“相对于”。例如，所有整数的集合$\mathbf{Z}$是一个关于加法而不是关于乘法的群(除了\pm 1之外，它没有其他元素的逆)。同样，集合$G={1，-1}$是一个关于乘法而不是关于加法的群。然而，在大多数情况下，只考虑一个二进制操作，我们简单地说“$G$是一个组”。如果未指定二进制操作，则采用乘法表示法，并使用并列式$x y$来表示$x$和$y$组合的结果。但是请记住，二进制操作不一定是乘法。

数学代写|现代代数代写Modern Algebra代考|Properties of Group Elements

群定义的几个结果记录在定理3.4中。
下一个定理的$\mathbf{a}$和$\mathbf{b}$部分是关于唯一性的陈述，它们可以用标准类型的唯一性证明来证明:假设存在两个这样的量，然后证明这两个量相等。
群元素的性质
设$G$是一个关于二进制运算的组，它被写成乘法。
a.“$G$”中的“$e$”是唯一的标识元素。
b.对于每个$x \in G$, $G$的倒数$x^{-1}$是唯一的。
c.对于每个$x \in G,\left(x^{-1}\right)^{-1}=x$。
d.逆序定律。有关任何$x$和$y$，请参阅$G,(x y)^{-1}=y^{-1} x^{-1}$。
e.取消法。如果$a, x$和$y$在$G$中，则公式$a x=a y$或$x a=y a$中的任何一个都意味着$x=y$。

我们证明了$\mathbf{b}$和$\mathbf{d}$部分，其余部分作为练习。为了证明部分$\mathbf{b}$，设$x \in G$，并假设$y$和$z$都是$x$的逆。也就是说，
$$
x y=e=y x \text { and } x z=e=z x .
$$
然后
$$
\begin{aligned}
y & =e y & & \text { since } e \text { is an identity } \
& =(z x) y & & \text { since } z x=e \
& =z(x y) & & \text { by associativity } \
& =z(e) & & \text { since } x y=e \
& =z & & \text { since } e \text { is an identity. }
\end{aligned}
$$
因此是$y=z$，这证明了将$x^{-1}$标记为$G$中$x$的唯一逆表示。
我们将在$\mathbf{d}$的证明中使用$\mathbf{b}$部分。具体来说，我们将利用逆$(x y)^{-1}$是唯一的这一事实。这意味着为了证明$y^{-1} x^{-1}=(x y)^{-1}$，我们只需要验证$(x y)\left(y^{-1} x^{-1}\right)=e=\left(y^{-1} x^{-1}\right)(x y)$。这些计算很简单:
$$
\left(y^{-1} x^{-1}\right)(x y)=y^{-1}\left(x^{-1} x\right) y=y^{-1} e y=y^{-1} y=e
$$
和
$$
(x y)\left(y^{-1} x^{-1}\right)=x\left(y y^{-1}\right) x^{-1}=x e x^{-1}=x x^{-1}=e .
$$

数学代写|现代代数代写Modern Algebra代考请认准statistics-lab™

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Prime Factors and Greatest Common Divisor

Posted on 2023年7月13日2023年7月13日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Prime Factors and Greatest Common Divisor

In this section, we establish the existence of the greatest common divisor of two integers when at least one of them is nonzero. The Unique Factorization Theorem, also known as the Fundamental Theorem of Arithmetic, is obtained.
Greatest Common Divisor
An integer $d$ is a greatest common divisor of $a$ and $b$ if all these conditions are satisfied:

$d$ is a positive integer.
$d \mid a$ and $d \mid b$.
$c \mid a$ and $c \mid b$ imply $c \mid d$.

The next theorem shows that the greatest common divisor $d$ of $a$ and $b$ exists when at least one of them is not zero. Our proof also shows that $d$ is a linear combination of $a$ and $b$; that is, $d=m a+n b$ for integers $m$ and $n$.

Let $a$ and $b$ be integers, at least one of them not 0 . Then there exists a unique greatest common divisor $d$ of $a$ and $b$. Moreover, $d$ can be written as
$$
d=a m+b n
$$
for integers $m$ and $n$, and $d$ is the smallest positive integer that can be written in this form.
Proof Let $a$ and $b$ be integers, at least one of them not 0 . If $b=0$, then $a \neq 0$, so $|a|>0$. It is easy to see that $d=|a|$ is a greatest common divisor of $a$ and $b$ in this case, and either $d=a \cdot(1)+b \cdot(0)$ or $d=a \cdot(-1)+b \cdot(0)$.

Suppose now that $b \neq 0$. Consider the set $S$ of all integers that can be written in the form $a x+$ by for some integers $x$ and $y$, and let $S^{+}$be the set of all positive integers in $S$. The set $S$ contains $b=a \cdot(0)+b \cdot(1)$ and $-b=a \cdot(0)+b \cdot(-1)$, so $S^{+}$is not empty. By the Well-Ordering Theorem, $S^{+}$has a least element $d$,
$$
d=a m+b n .
$$
We have $d$ positive, and we shall show that $d$ is a greatest common divisor of $a$ and $b$.
By the Division Algorithm, there are integers $q$ and $r$ such that
$$
a=d q+r \text { with } 0 \leq r<d .
$$
From this equation,
$$
\begin{aligned}
r & =a-d q \
& =a-(a m+b n) q \
& =a(1-m q)+b(-n q) .
\end{aligned}
$$

数学代写|现代代数代写Modern Algebra代考|The Euclidean Algorithm

$$
\begin{array}{rlrl}
a & =b q_0+r_1, & 0 \leq r_1<b \
b & =r_1 q_1+r_2, & 0 \leq r_2<r_1 \
r_1 & =r_2 q_2+r_3, & 0 \leq r_3<r_2 \
\vdots & & \vdots \
r_k & =r_{k+1} q_{k+1}+r_{k+2}, & & 0 \leq r_{k+2}<r_{k+1} .
\end{array}
$$
Since the integers $r_1, r_2, \ldots, r_{k+2}$ are decreasing and are all nonnegative, there is a smallest integer $n$ such that $r_{n+1}=0$ :
$$
r_{n-1}=r_n q_n+r_{n+1}, \quad 0=r_{n+1} .
$$
If we put $r_0=b$, this last nonzero remainder $r_n$ is always the greatest common divisor of $a$ and $b$. The proof of this statement is left as an exercise.
As an example, we shall find the greatest common divisor of 1492 and 1776.
Example 1 Performing the arithmetic for the Euclidean Algorithm, we have
$$
\begin{aligned}
1776 & =(1)(1492)+\mathbf{2 8 4} & & \left(q_0=1, r_1=284\right) \
1492 & =(5)(\mathbf{2 8 4})+\mathbf{7 2} & & \left(q_1=5, r_2=72\right) \
\mathbf{2 8 4} & =(3)(\mathbf{7 2})+\mathbf{6 8} & & \left(q_2=3, r_3=68\right) \
\mathbf{7 2} & =(1)(\mathbf{6 8})+\mathbf{4} & & \left(q_3=1, r_4=4\right) \
\mathbf{6 8} & =(\mathbf{4})(17) & & \left(q_4=17, r_5=0\right) .
\end{aligned}
$$
Thus the last nonzero remainder is $r_n=r_4=4$, and $(1776,1492)=4$.

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Prime Factors and Greatest Common Divisor

在本节中，当两个整数中至少有一个非零时，我们建立了它们最大公约数的存在性。得到了唯一因数分解定理，又称算术基本定理。
最大公约数
如果满足所有这些条件，整数$d$是$a$和$b$的最大公约数:

$d$ 是一个正整数。

$d \mid a$ 还有$d \mid b$。

$c \mid a$$c \mid b$暗示$c \mid d$。

下一个定理表明，当$a$和$b$至少有一个不为零时，存在最大公约数$d$。我们的证明还表明$d$是$a$和$b$的线性组合;即对于整数$m$和$n$，为$d=m a+n b$。

设$a$和$b$为整数，其中至少有一个不为0。那么$a$和$b$存在唯一的最大公约数$d$。此外，$d$可以写成
$$
d=a m+b n
$$
对于整数$m$和$n$, $d$是可以写成这种形式的最小的正整数。
证明设$a$和$b$为整数，且至少有一个不为0。如果$b=0$，那么$a \neq 0$，那么$|a|>0$。很容易看出，在这种情况下，$d=|a|$是$a$和$b$的最大公约数，$d=a \cdot(1)+b \cdot(0)$或$d=a \cdot(-1)+b \cdot(0)$也是如此。

现在假设$b \neq 0$。考虑所有整数的集合$S$，这些整数可以写成$a x+$的形式，对于某些整数$x$和$y$，设$S^{+}$为$S$中所有正整数的集合。集合$S$包含$b=a \cdot(0)+b \cdot(1)$和$-b=a \cdot(0)+b \cdot(-1)$，因此$S^{+}$不是空的。根据良序定理，$S^{+}$有一个最小元素$d$，
$$
d=a m+b n .
$$
我们有$d$是正数，我们将证明$d$是$a$和$b$的最大公约数。
通过除法算法，存在整数$q$和$r$，使得
$$
a=d q+r \text { with } 0 \leq r<d .
$$
由这个方程，
$$
\begin{aligned}
r & =a-d q \
& =a-(a m+b n) q \
& =a(1-m q)+b(-n q) .
\end{aligned}
$$

数学代写|现代代数代写Modern Algebra代考|The Euclidean Algorithm

$$
\begin{array}{rlrl}
a & =b q_0+r_1, & 0 \leq r_1<b \
b & =r_1 q_1+r_2, & 0 \leq r_2<r_1 \
r_1 & =r_2 q_2+r_3, & 0 \leq r_3<r_2 \
\vdots & & \vdots \
r_k & =r_{k+1} q_{k+1}+r_{k+2}, & & 0 \leq r_{k+2}<r_{k+1} .
\end{array}
$$
由于整数$r_1, r_2, \ldots, r_{k+2}$都是递减的且都是非负的，因此存在一个最小整数$n$，使得$r_{n+1}=0$:
$$
r_{n-1}=r_n q_n+r_{n+1}, \quad 0=r_{n+1} .
$$
如果我们代入$r_0=b$，最后一个非零余数$r_n$总是$a$和$b$的最大公约数。这句话的证明留作练习。
作为一个例子，我们将找出1492和1776的最大公约数。
例1执行欧几里得算法的算术，我们有
$$
\begin{aligned}
1776 & =(1)(1492)+\mathbf{2 8 4} & & \left(q_0=1, r_1=284\right) \
1492 & =(5)(\mathbf{2 8 4})+\mathbf{7 2} & & \left(q_1=5, r_2=72\right) \
\mathbf{2 8 4} & =(3)(\mathbf{7 2})+\mathbf{6 8} & & \left(q_2=3, r_3=68\right) \
\mathbf{7 2} & =(1)(\mathbf{6 8})+\mathbf{4} & & \left(q_3=1, r_4=4\right) \
\mathbf{6 8} & =(\mathbf{4})(17) & & \left(q_4=17, r_5=0\right) .
\end{aligned}
$$
因此最后的非零余数是$r_n=r_4=4$和$(1776,1492)=4$。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Sets

Posted on 2023年7月13日2023年7月13日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Sets

Abstract algebra had its beginnings in attempts to address mathematical problems such as the solution of polynomial equations by radicals and geometric constructions with straightedge and compass. From the solutions of specific problems, general techniques evolved that could be used to solve problems of the same type, and treatments were generalized to deal with whole classes of problems rather than individual ones.

In our study of abstract algebra, we shall make use of our knowledge of the various number systems. At the same time, in many cases we wish to examine how certain properties are consequences of other, known properties. This sort of examination deepens our understanding of the system. As we proceed, we shall be careful to distinguish between the properties we have assumed and made available for use and those that must be deduced from these properties. We must accept without definition some terms that are basic objects in our mathematical systems. Initial assumptions about each system are formulated using these undefined terms.

One such undefined term is set. We think of a set as a collection of objects about which it is possible to determine whether or not a particular object is a member of the set. Sets are usually denoted by capital letters and are sometimes described by a list of their elements, as illustrated in the following examples.

数学代写|现代代数代写Modern Algebra代考|Mappings

The concept of a function is fundamental to nearly all areas of mathematics. The term function is the one most widely used for the concept that we have in mind, but it has become traditional to use the terms mapping and transformation in algebra. It is likely that these words are used because they express an intuitive feel for the association between the elements involved. The basic idea is that correspondences of a certain type exist between the elements of two sets. There is to be a rule of association between the elements of a first set and those of a second set. The association is to be such that for each element in the first set, there is one and only one associated element in the second set. This rule of association leads to a natural pairing of the elements that are to correspond, and then to the formal statement in Definition 1.9.

By an ordered pair of elements we mean a pairing $(a, b)$, where there is to be a distinction between the pair $(a, b)$ and the pair $(b, a)$, if $a$ and $b$ are different. That is, there is to be a first position and a second position such that $(a, b)=(c, d)$ if and only if both $a=c$ and $b=d$. This ordering is altogether different from listing the elements of a set, for there the order of listing is of no consequence at all. The sets ${1,2}$ and ${2,1}$ have exactly the same elements, and ${1,2}={2,1}$. When we speak of ordered pairs, however, we do not consider $(1,2)$ and $(2,1)$ equal. With these ideas in mind, we make the following definition.

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Sets

抽象代数的起源是试图解决数学问题，比如用根式解多项式方程，用直尺和指南针构造几何结构。从特定问题的解决方案，发展出可用于解决同一类型问题的通用技术，并且处理方法被一般化以处理整个类别的问题，而不是单个问题。

在学习抽象代数时，我们将利用各种数制的知识。同时，在许多情况下，我们希望研究某些属性是如何由其他已知属性导致的。这种考察加深了我们对制度的理解。在我们继续进行的过程中，我们将仔细区分我们已经假定并可供使用的性质和那些必须从这些性质中推导出来的性质。我们必须不加定义地接受一些术语，它们是我们数学系统中的基本对象。每个系统的初始假设是用这些未定义的术语来表述的。

其中一个未定义的术语就是set。我们认为集合是一组对象的集合，通过这些对象可以确定某个特定对象是否为集合的成员。集合通常用大写字母表示，有时用其元素的列表来描述，如下面的例子所示。

数学代写|现代代数代写Modern Algebra代考|Mappings

函数的概念是几乎所有数学领域的基础。函数这个术语是我们脑海中使用最广泛的概念，但是在代数中使用映射和变换已经成为传统。使用这些词很可能是因为它们表达了对相关元素之间联系的直观感觉。其基本思想是两个集合的元素之间存在某种类型的对应关系。在第一个集合的元素和第二个集合的元素之间必须有一个关联规则。这种关联是这样的:对于第一个集合中的每个元素，在第二个集合中有且只有一个关联元素。这个关联规则导致要对应的元素的自然配对，然后是定义1.9中的形式声明。

我们所说的有序元素对是指一对$(a, b)$，如果$a$和$b$不同，则对$(a, b)$和对$(b, a)$之间是有区别的。也就是说，存在第一位置和第二位置，使得$(a, b)=(c, d)$当且仅当$a=c$和$b=d$。这种排序与列出集合的元素完全不同，因为列出的顺序根本无关紧要。集合${1,2}$和${2,1}$具有完全相同的元素，并且${1,2}={2,1}$。然而，当我们谈到有序对时，我们不认为$(1,2)$和$(2,1)$相等。考虑到这些想法，我们做出以下定义。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Mignotte’s factor bound and a modular gcd algorithm in Z[x]

Posted on 2023年6月29日2023年6月29日 by statistics-lab

如果你也在怎样代写现代代数Modern Algebra 这个学科遇到相关的难题，请随时右上角联系我们的24/7代写客服。现代代数Modern Algebra现代代数，也叫抽象代数，是数学的一个分支，涉及各种集合（如实数、复数、矩阵和矢量空间）的一般代数结构，而不是操作其个别元素的规则和程序。除了数论和代数几何的发展，现代代数通过群论对对称性有重要的应用。群这个词通常指的是一组运算，可能保留了某些物体的对称性或类似物体的排列。

现代代数Modern Algebra代数是数学的一个分支的名称，但它也是一种数学结构的名称。代数或代数结构是一个带有运算的非空集合。从一般结构角度研究代数的数学分支被称为普遍代数。相比之下，现代代数处理的是特殊类别的代数，包括群、环、场、向量空间和模块。从普遍代数的角度来看，场、向量空间和模块不被视为代数结构。现代代数也被称为抽象代数，但这两个名字在今天都有误导性，因为它在现代数学中已经不怎么现代或抽象了。

数学代写|现代代数代写Modern Algebra代考|Mignotte’s factor bound and a modular gcd algorithm in Z[x]

In order to adapt Algorithm 6.28 to $\mathbb{Z}[x]$, we need an a priori bound on the coefficient size of $h$. Over $F[y]$, the bound
$$
\operatorname{deg}_y h \leq \operatorname{deg}_y f
$$
is trivial and quite sufficient. Over $\mathbb{Z}$, we could use the subresultant bound of Theorem 6.52 below, but we now derive a much better bound. It actually depends only on one argument of the gcd, say $f$, and is valid for all factors of $f$. We will use this again for the factorization of $f$ in Chapter 15 .

We extend the 2-norm to a complex polynomial $f=\sum_{0 \leq i \leq n} f_i x^i \in \mathbb{C}[x]$ by $|f|_2=\left(\sum_{0 \leq i \leq n}\left|f_i\right|^2\right)^{1 / 2} \in \mathbb{R}$, where $|a|=(a \cdot \bar{a})^{1 / 2} \in \mathbb{R}$ is the norm of $a \in \mathbb{C}$ and $\bar{a}$ is the complex conjugate of $a$. We will derive a bound for the norm of factors of $f$ in terms of $|f|_2$, that is, a bound $B \in \mathbb{R}$ such that any factor $h \in \mathbb{Z}[x]$ of $f$ satisfies $|h|_2 \leq B$. One might hope that we can take $B=|f|_2$, but this is not the case. For example, let $f=x^n-1$ and $h=\Phi_n \in \mathbb{Z}[x]$ be the $n$th cyclotomic polynomial (Section 14.10). Thus $\Phi_n$ divides $x^n-1$, and the direct analog of (8) would say that each coefficient of $\Phi_n$ is at most 1 in absolute value, but for example $\Phi_{105}$, of degree 48 , contains the term $-2 x^7$. In fact, the coefficients of $\Phi_n$ are unbounded in absolute value if $n \longrightarrow \infty$, and hence this is also true for $|h|_2$. Worse yet, for infinitely many integers $n, \Phi_n$ has a very large coefficient, namely larger than $\exp (\exp (\ln 2 \cdot \ln n / \ln \ln n))$, where $\ln$ is the logarithm in base $e$; such a coefficient has word length somewhat less than $n$. It is not obvious how to control the coefficients of factors at all, and it is not surprising that we have to work a little bit to establish a good bound.

数学代写|现代代数代写Modern Algebra代考|Small primes modular gcd algorithms

We have seen in Section 5.5 that the small primes modular approach for computing the determinant is computationally superior to the big prime scheme. The reason that we have discussed big prime modular gcd algorithms at all in the preceding sections is that they are easier and the main idea is more clearly visible than for their small prime variants that we will present now. In practice, we strongly recommend the use of the latter. We start with the algorithm for $F[x, y]$ since it is simpler to describe and analyze than the corresponding algorithm for $\mathbb{Z}[x]$.
AlgORITHM 6.36 Modular bivariate ged: small primes version.
Input: Primitive polynomials $f, g \in F[x, y]=R[x]$ with $\operatorname{deg}_x f=n \geq \operatorname{deg}_x g \geq 1$ and $\operatorname{deg}_y f, \operatorname{deg}_y g \leq d$, where $R=F[y]$ for a field $F$ with at least $(4 n+2) d$ elements. Output: $h=\operatorname{gcd}(f, g) \in R[x]$.

$b \longleftarrow \operatorname{gcd}\left(\operatorname{lc}_x(f), \operatorname{lc}_x(g)\right), \quad l \longleftarrow d+1+\operatorname{deg}_y b$

repeat

choose a set $S \subseteq F$ of $2 l$ evaluation points

$S \longleftarrow{u \in S: b(u) \neq 0}$
for each $u \in S$ call the Euclidean Algorithm 3.14 over $F$ to compute the monic $v_u=\operatorname{gcd}(f(x, u), g(x, u)) \in F[x]$

$e \longleftarrow \min \left{\operatorname{deg} v_u: u \in S\right}, \quad S \longleftarrow\left{u \in S: \operatorname{deg} v_u=e\right}$ if $# S \geq l$ then remove $# S-l$ elements from $S$ else goto 3

compute by interpolation each coefficient in $F[y]$ of the polynomials $w, f^, g^ \in R[x]$ of degrees in $y$ less than $l$ such that
$$
w(x, u)=b(u) v_u
$$

$$
f^(x, u) w(x, u)=b(u) f(x, u), \quad g^(x, u) w(x, u)=b(u) g(x, u)
$$
for all $u \in S$

until $\operatorname{deg}_y\left(f^* w\right)=\operatorname{deg}_y(b f)$ and $\operatorname{deg}_y\left(g^* w\right)=\operatorname{deg}_y(b g)$

return $\mathrm{pp}_x(w)$

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Mignotte’s factor bound and a modular gcd algorithm in Z[x]

为了使算法6.28适应$\mathbb{Z}[x]$，我们需要对$h$的系数大小有一个先验的界。除以$F[y]$，边界
$$
\operatorname{deg}_y h \leq \operatorname{deg}_y f
$$
是微不足道的，而且是足够的。在$\mathbb{Z}$上，我们可以使用下面定理6.52的次结界，但我们现在推导出一个更好的界。它实际上只依赖于gcd的一个参数，比如$f$，并且对$f$的所有因素都有效。我们将在第15章中再次使用它来分解$f$。

我们通过$|f|2=\left(\sum{0 \leq i \leq n}\left|f_i\right|^2\right)^{1 / 2} \in \mathbb{R}$将2范数扩展到一个复多项式$f=\sum_{0 \leq i \leq n} f_i x^i \in \mathbb{C}[x]$，其中$|a|=(a \cdot \bar{a})^{1 / 2} \in \mathbb{R}$是$a \in \mathbb{C}$的范数，$\bar{a}$是$a$的复共轭。我们将用$|f|2$来推导$f$的因子范数的一个界，即，一个界$B \in \mathbb{R}$使得$f$的任何因子$h \in \mathbb{Z}[x]$满足$|h|_2 \leq B$。有人可能希望我们可以采取$B=|f|_2$，但事实并非如此。例如，设$f=x^n-1$和$h=\Phi_n \in \mathbb{Z}[x]$是$n$的第一个分环多项式(第14.10节)。因此$\Phi_n$除$x^n-1$，与(8)的直接类比会说，$\Phi_n$的每个系数的绝对值最多为1，但例如，次为48的$\Phi{105}$包含了$-2 x^7$项。事实上，$\Phi_n$的系数在$n \longrightarrow \infty$的绝对值上是无界的，因此对于$|h|_2$也是如此。更糟糕的是，对于无穷多个整数$n, \Phi_n$有一个非常大的系数，即大于$\exp (\exp (\ln 2 \cdot \ln n / \ln \ln n))$，其中$\ln$是以$e$为底的对数;该系数的字长略小于$n$。如何控制因子的系数一点也不明显，所以我们需要花点功夫来建立一个好的界也就不足为奇了。

数学代写|现代代数代写Modern Algebra代考|Small primes modular gcd algorithms

在第5.5节中我们已经看到，计算行列式的小素数模块化方法在计算上优于大素数方案。我们在前面几节中讨论大素数模块化gcd算法的原因是，它们比我们现在要介绍的小素数变体更容易，而且主要思想更清晰可见。在实践中，我们强烈建议使用后者。我们从$F[x, y]$的算法开始，因为它比$\mathbb{Z}[x]$的相应算法更容易描述和分析。
算法6.36模二元格:小素数版本。
输入:包含$\operatorname{deg}_x f=n \geq \operatorname{deg}_x g \geq 1$和$\operatorname{deg}_y f, \operatorname{deg}_y g \leq d$的原语多项式$f, g \in F[x, y]=R[x]$，其中$R=F[y]$表示包含至少$(4 n+2) d$个元素的字段$F$。输出:$h=\operatorname{gcd}(f, g) \in R[x]$。

$b \longleftarrow \operatorname{gcd}\left(\operatorname{lc}_x(f), \operatorname{lc}_x(g)\right), \quad l \longleftarrow d+1+\operatorname{deg}_y b$

重复

选择一组$S \subseteq F$的$2 l$评估点

$S \longleftarrow{u \in S: b(u) \neq 0}$
对于每个$u \in S$调用欧几里得算法3.14除以$F$来计算monic $v_u=\operatorname{gcd}(f(x, u), g(x, u)) \in F[x]$

$e \longleftarrow \min \left{\operatorname{deg} v_u: u \in S\right}, \quad S \longleftarrow\left{u \in S: \operatorname{deg} v_u=e\right}$ 如果是$# S \geq l$，则从$S$中删除$# S-l$元素，否则转到3

通过插值计算中的每个系数 $F[y]$ 关于多项式的 $w, f^, g^ \in R[x]$ 学位的 $y$ 小于 $l$ 这样
$$
w(x, u)=b(u) v_u
$$

$$
f^(x, u) w(x, u)=b(u) f(x, u), \quad g^(x, u) w(x, u)=b(u) g(x, u)
$$
对所有人 $u \in S$

直到$\operatorname{deg}_y\left(f^* w\right)=\operatorname{deg}_y(b f)$和 $\operatorname{deg}_y\left(g^* w\right)=\operatorname{deg}_y(b g)$

返回 $\mathrm{pp}_x(w)$

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

金融工程是使用数学技术来解决金融问题。金融工程使用计算机科学、统计学、经济学和应用数学领域的工具和知识来解决当前的金融问题，以及设计新的和创新的金融产品。

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

术语广义线性模型（GLM）通常是指给定连续和/或分类预测因素的连续响应变量的常规线性回归模型。它包括多元线性回归，以及方差分析和方差分析（仅含固定效应）。

有限元方法代写

有限元方法（FEM）是一种流行的方法，用于数值解决工程和数学建模中出现的微分方程。典型的问题领域包括结构分析、传热、流体流动、质量运输和电磁势等传统领域。

有限元是一种通用的数值方法，用于解决两个或三个空间变量的偏微分方程（即一些边界值问题）。为了解决一个问题，有限元将一个大系统细分为更小、更简单的部分，称为有限元。这是通过在空间维度上的特定空间离散化来实现的，它是通过构建对象的网格来实现的：用于求解的数值域，它有有限数量的点。边界值问题的有限元方法表述最终导致一个代数方程组。该方法在域上对未知函数进行逼近。[1] 然后将模拟这些有限元的简单方程组合成一个更大的方程系统，以模拟整个问题。然后，有限元通过变化微积分使相关的误差函数最小化来逼近一个解决方案。

tatistics-lab作为专业的留学生服务机构，多年来已为美国、英国、加拿大、澳洲等留学热门地的学生提供专业的学术服务，包括但不限于Essay代写，Assignment代写，Dissertation代写，Report代写，小组作业代写，Proposal代写，Paper代写，Presentation代写，计算机作业代写，论文修改和润色，网课代做，exam代考等等。写作范围涵盖高中，本科，研究生等海外留学全阶段，辐射金融，经济学，会计学，审计学，管理学等全球99%专业科目。写作团队既有专业英语母语作者，也有海外名校硕博留学生，每位写作老师都拥有过硬的语言能力，专业的学科背景和学术写作经验。我们承诺100%原创，100%专业，100%准时，100%满意。

随机分析代写

随机微积分是数学的一个分支，对随机过程进行操作。它允许为随机过程的积分定义一个关于随机过程的一致的积分理论。这个领域是由日本数学家伊藤清在第二次世界大战期间创建并开始的。

时间序列分析代写

随机过程，是依赖于参数的一组随机变量的全体，参数通常是时间。随机变量是随机现象的数量表现，其时间序列是一组按照时间发生先后顺序进行排列的数据点序列。通常一组时间序列的时间间隔为一恒定值（如1秒，5分钟，12小时，7天，1年），因此时间序列可以作为离散时间数据进行分析处理。研究时间序列数据的意义在于现实中，往往需要研究某个事物其随时间发展变化的规律。这就需要通过研究该事物过去发展的历史记录，以得到其自身发展的规律。

回归分析代写

多元回归分析渐进（Multiple Regression Analysis Asymptotics）属于计量经济学领域，主要是一种数学上的统计分析方法，可以分析复杂情况下各影响因素的数学关系，在自然科学、社会和经济学等多个领域内应用广泛。

MATLAB代写

MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中，其中问题和解决方案以熟悉的数学符号表示。典型用途包括：数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发，包括图形用户界面构建MATLAB 是一个交互式系统，其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题，尤其是那些具有矩阵和向量公式的问题，而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问，这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展，得到了许多用户的投入。在大学环境中，它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域，MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要，工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数（M 文件）的综合集合，可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Partial fraction decomposition

Posted on 2023年6月29日2023年6月29日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Partial fraction decomposition

We discuss another one of the numerous applications of the Chinese Remainder Theorem for polynomials. It will be put to use in Chapter 22 .

Let $F$ be a field, $f_1, \ldots, f_r \in F[x]$ nonconstant monic and pairwise coprime polynomials, $e_1, \ldots, e_r \in \mathbb{N}$ positive integers, and $f=f_1^{e_1} \cdots f_r^{e_r}$. (We will see in Part III how to factor polynomials over finite fields and over $\mathbb{Q}$ into irreducible factors, but here we do not assume irreducibility of the $f_i$.) For another polynomial $g \in F[x]$ of degree less than $n=\operatorname{deg} f$, the partial fraction decomposition of the rational function $g / f \in F(x)$ with respect to the given factorization of the denominator $f$ is
$$
\frac{g}{f}=\frac{g_{1,1}}{f_1}+\cdots+\frac{g_{1, e_1}}{f_1^{e_1}}+\cdots+\frac{g_{r, 1}}{f_r}+\cdots+\frac{g_{r, e_r}}{f_r^{e_r}},
$$
with $g_{i j} \in F[x]$ of smaller degree than $f_i$, for all $i, j$. If all $f_i$ are linear polynomials, then the $g_{i j}$ are just constants.

EXAMPLE 5.28. Let $F=\mathbb{Q}, f=x^4-x^2$, and $g=x^3+4 x^2-x-2$. The partial fraction decomposition of $g / f$ with respect to the factorization $f=x^2(x-1)(x+1)$ of $f$ into linear polynomials is
$$
\frac{x^3+4 x^2-x-2}{x^4-x^2}=\frac{1}{x}+\frac{2}{x^2}+\frac{1}{x-1}+\frac{-1}{x+1}
$$
The following questions pose themselves: Does a decomposition as in (31) always exist uniquely, and how can we compute it? The next lemma is a first step towards an answer.

数学代写|现代代数代写Modern Algebra代考|Coefficient growth in the Euclidean Algorithm

Let $F$ be a field, and $f, g \in F[x]$ with $\operatorname{deg} f=n \geq \operatorname{deg} g=m \geq 0$. We fix the notation from Section 3.4 of the results of the Extended Euclidean Algorithm for $f$ and $g$ :
$$
\begin{aligned}
& \rho_0 r_0=f \
& \rho_0 s_0=1 \text {, } \
& \rho_0 t_0=0, \
& \rho_1 r_1=g \
& \rho_1 s_1=0 \text {, } \
& \rho_1 t_1=1 \text {, } \
& \rho_2 r_2=r_0-q_1 r_1 \text {, } \
& \rho_2 s_2=s_0-q_1 s_1 \text {, } \
& \rho_2 t_2=t_0-q_1 t_1 \text {, } \
& \vdots \
& \vdots \
& \text { : } \
& \rho_{i+1} r_{i+1}=r_{i-1}-q_i r_i, \quad \rho_{i+1} s_{i+1}=s_{i-1}-q_i s_i, \quad \rho_{i+1} t_{i+1}=t_{i-1}-q_i t_i, \
& \vdots \
& 0=r_{\ell-1}-q_{\ell} r_{\ell}, \
& \vdots \
& \text { : } \
& s_{\ell+1}=s_{\ell-1}-q_{\ell} s_{\ell}, \
& t_{\ell+1}=t_{\ell-1}-q_{\ell} t_{\ell}, \
&
\end{aligned}
$$

with $\operatorname{deg} r_{i+1}<\operatorname{deg} r_i$ for all $i \geq 1$. Thus $r_{i-1}=q_i r_i+\rho_{i+1} r_{i+1}$ is the division of $r_{i-1}$ by $r_i$ with remainder $\rho_{i+1} r_{i+1}$; the leading coefficient $\rho_{i+1}$ serves to have a normalized remainder $r_{i+1}$. A basic invariant is $r_i=s_i f+t_i g$. We define the degree sequence $\left(n_0, n_1, \ldots, n_{\ell}\right)$ by $n_i=\operatorname{deg} r_i$ for all $i$. Then $$ n=n_0 \geq n_1>n_2 \cdots>n_{\ell} \geq 0 .
$$
It is convenient to set $\rho_{\ell+1}=1, r_{\ell+1}=0$, and $n_{\ell+1}=-\infty$. The number of arithmetic operations in $F$ performed by the (Extended) Euclidean Algorithm for $f$ and $g$ is $O(n m)$ (Theorem 3.16).

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Partial fraction decomposition

我们讨论多项式的中国剩余定理的众多应用中的另一个。它将在第22章中使用。

设$F$为一个域，$f_1, \ldots, f_r \in F[x]$为非常一元多项式和对素数多项式，$e_1, \ldots, e_r \in \mathbb{N}$为正整数，$f=f_1^{e_1} \cdots f_r^{e_r}$为正整数。(我们将在第三部分看到如何将有限域和$\mathbb{Q}$上的多项式分解为不可约因子，但在这里我们不假设$f_i$不可约。)对于另一个次小于$n=\operatorname{deg} f$的多项式$g \in F[x]$，有理函数$g / f \in F(x)$相对于给定的分母$f$的因式分解的部分分式分解为
$$
\frac{g}{f}=\frac{g_{1,1}}{f_1}+\cdots+\frac{g_{1, e_1}}{f_1^{e_1}}+\cdots+\frac{g_{r, 1}}{f_r}+\cdots+\frac{g_{r, e_r}}{f_r^{e_r}},
$$
对于所有$i, j$, $g_{i j} \in F[x]$的度数小于$f_i$。如果所有的$f_i$都是线性多项式，那么$g_{i j}$就是常数。

例5.28。设$F=\mathbb{Q}, f=x^4-x^2$和$g=x^3+4 x^2-x-2$。$g / f$对于$f$的因式分解$f=x^2(x-1)(x+1)$的部分分式分解为线性多项式为
$$
\frac{x^3+4 x^2-x-2}{x^4-x^2}=\frac{1}{x}+\frac{2}{x^2}+\frac{1}{x-1}+\frac{-1}{x+1}
$$
下面的问题提出了:(31)中的分解是否总是唯一存在，我们如何计算它?下一个引理是找到答案的第一步。

数学代写|现代代数代写Modern Algebra代考|Coefficient growth in the Euclidean Algorithm

设$F$为字段，$f, g \in F[x]$为$\operatorname{deg} f=n \geq \operatorname{deg} g=m \geq 0$。我们对$f$和$g$的扩展欧几里得算法结果的3.4节中的符号进行了修正:
$$
\begin{aligned}
& \rho_0 r_0=f \
& \rho_0 s_0=1 \text {, } \
& \rho_0 t_0=0, \
& \rho_1 r_1=g \
& \rho_1 s_1=0 \text {, } \
& \rho_1 t_1=1 \text {, } \
& \rho_2 r_2=r_0-q_1 r_1 \text {, } \
& \rho_2 s_2=s_0-q_1 s_1 \text {, } \
& \rho_2 t_2=t_0-q_1 t_1 \text {, } \
& \vdots \
& \vdots \
& \text { : } \
& \rho_{i+1} r_{i+1}=r_{i-1}-q_i r_i, \quad \rho_{i+1} s_{i+1}=s_{i-1}-q_i s_i, \quad \rho_{i+1} t_{i+1}=t_{i-1}-q_i t_i, \
& \vdots \
& 0=r_{\ell-1}-q_{\ell} r_{\ell}, \
& \vdots \
& \text { : } \
& s_{\ell+1}=s_{\ell-1}-q_{\ell} s_{\ell}, \
& t_{\ell+1}=t_{\ell-1}-q_{\ell} t_{\ell}, \
&
\end{aligned}
$$

有 $\operatorname{deg} r_{i+1}<\operatorname{deg} r_i$ 对所有人 $i \geq 1$．因此 $r_{i-1}=q_i r_i+\rho_{i+1} r_{i+1}$ 的除法 $r_{i-1}$ 通过 $r_i$ 带余数 $\rho_{i+1} r_{i+1}$；前导系数 $\rho_{i+1}$ 有一个归一化余数 $r_{i+1}$．一个基本不变式是 $r_i=s_i f+t_i g$．我们定义度序列 $\left(n_0, n_1, \ldots, n_{\ell}\right)$ 通过 $n_i=\operatorname{deg} r_i$ 对所有人 $i$．然后 $$ n=n_0 \geq n_1>n_2 \cdots>n_{\ell} \geq 0 .
$$
设置方便 $\rho_{\ell+1}=1, r_{\ell+1}=0$，和 $n_{\ell+1}=-\infty$．中的算术运算次数 $F$ 由(扩展的)欧几里得算法执行 $f$ 和 $g$ 是 $O(n m)$ (定理3.16)。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

有限元方法代写

随机分析代写

时间序列分析代写

回归分析代写

MATLAB代写

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Application: Secret sharing

Posted on 2023年6月29日2023年6月29日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Application: Secret sharing

A neat application of interpolation, which we mentioned in Section 1.3 but which will not be used later, is secret sharing: you want to give to $n$ players a shared secret, so that together they can discover it, but no proper subset of the players can. To achieve this, you identify possible secrets with elements of the finite field $\mathbb{F}_p=\mathbb{Z} /\langle p\rangle$ for an appropriate $p$. Some bank cards for Automatic Teller Machine access have as their secret PIN codes four-digit decimal numbers. For such a secret, you choose a prime $p$ just bigger than 10000 , say $p=10007$. Then you choose $2 n-1$ random elements $f_1, \ldots, f_{n-1}, u_0, \ldots, u_{n-1} \in \mathbb{F}p$ uniformly and independently with all $u_i$ nonzero, call your secret $f_0$, set $f=f{n-1} x^{n-1}+\cdots+f_1 x+f_0 \in$ $\mathbb{F}_p[x]$, and give to player number $i$ the value $f\left(u_i\right) \in \mathbb{F}_p$. (If $u_i=u_j$ for some $i \neq j$, you have to make a new random choice; this is unlikely to happen if $n \ll \sqrt{p}$.) Then together they can determine the (unique) interpolation polynomial $f$ of degree less than $n$, and thus $f_0$. But if any smaller number of them, say $n-1$, get together, then the possible interpolation polynomials consistent with this partial knowledge are such that each value in $\mathbb{F}_p$ of $f_0$ is equally likely: they have no information on $f_0$ (Exercise 5.14).

We can extend this scheme to the situation where $k \leq n$ and each subset of $k$ players are able to recover the secret, but no set of fewer than $k$ players can. This is achieved by randomly and independently choosing $n+k-1$ elements $u_0, \ldots, u_{n-1}, f_1, \ldots, f_{k-1} \in \mathbb{F}p$ and giving $f\left(u_i\right)$ to player $i$, where $f=f{k-1} x^{k-1}+$ $\cdots+f_1 x+f_0 \in \mathbb{F}_p[x]$ and $f_0 \in \mathbb{F}_p$ is the secret as above. Again, it is required that $u_i \neq u_j$ if $i \neq j$. Since $f$ is uniquely determined by its values at $k$ points, each subset of $k$ out of the $n$ players can calculate $f$ and thus the secret $f_0$, but fewer than $k$ players together have no information on $f_0$.

数学代写|现代代数代写Modern Algebra代考|The Chinese Remainder Algorithm

Suppose that $f \in \mathbb{N}$ has two decimal digits and has remainder 2 on division by 11 and 7 on division by 13 . Does this uniquely define $f$, and if so, is there a better way to find it than to check all values between 0 and 99 ? We will see in this section that the answer to both questions is positive.
For this section, $R$ is a Euclidean domain, and we fix the following notation:
$m_0, \ldots, m_{r-1} \in R$ are pairwise coprime, so that $\operatorname{gcd}\left(m_i, m_j\right)=1$
for $0 \leq i<j<r$, and $m=m_0 \cdots m_{r-1}$.
Thus $m=\operatorname{lcm}\left(m_0, \ldots, m_{r-1}\right)$. For $0 \leq i<r$, we have the canonical ring homomorphism
$$
\begin{aligned}
\pi_i: R & \longrightarrow R /\left\langle m_i\right\rangle, \
f & \longmapsto f \bmod m_i .
\end{aligned}
$$
Combining these for all $i$, we get the ring homomorphism
$$
\begin{aligned}
& \chi=\pi_0 \times \cdots \times \pi_{r-1}: R \longrightarrow R /\left\langle m_0\right\rangle \times \ldots \times R /\left\langle m_{r-1}\right\rangle, \
& f \longmapsto\left(f \bmod m_0, \ldots, f \bmod m_{r-1}\right) \text {. } \
&
\end{aligned}
$$
For our example above, we have $R=\mathbb{Z}, r=2, m_0=11, m_1=13, m=143$, and
$$
\chi(f)=(f \bmod 11, f \bmod 13)=(2 \bmod 11,7 \bmod 13) \in \mathbb{Z}{11} \times \mathbb{Z}{13} .
$$
The following statement provides, in somewhat abstract terminology, the theoretical basis for many of our algorithms.

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Application: Secret sharing

我们在1.3节中提到的插值的一个简洁应用是秘密共享:你想给$n$玩家一个共享的秘密，这样他们就可以一起发现它，但没有适当的玩家子集可以发现它。要实现这一点，需要使用对应$p$的有限域$\mathbb{F}p=\mathbb{Z} /\langle p\rangle$的元素来识别可能的秘密。一些用于自动柜员机的银行卡的密码为四位十进制数字。对于这样一个秘密，您选择一个质数$p$略大于10000，例如$p=10007$。然后，您选择$2 n-1$随机元素$f_1, \ldots, f{n-1}, u_0, \ldots, u_{n-1} \in \mathbb{F}p$一致和独立与所有$u_i$非零，调用您的秘密$f_0$，设置$f=f{n-1} x^{n-1}+\cdots+f_1 x+f_0 \in$$\mathbb{F}_p[x]$，并给玩家号码$i$的值$f\left(u_i\right) \in \mathbb{F}_p$。(如果$u_i=u_j$对于一些$i \neq j$，你必须做一个新的随机选择;这不大可能发生，如果$n \ll \sqrt{p}$。)然后它们一起可以确定(唯一的)次小于$n$的插值多项式$f$，从而确定$f_0$。但是，如果它们的数量更少，比如$n-1$，聚集在一起，那么与这个部分知识一致的可能插值多项式是这样的:$f_0$的$\mathbb{F}_p$中的每个值都是相等的:它们没有$f_0$的信息(练习5.14)。

我们可以将此方案扩展到$k \leq n$和$k$玩家的每个子集都能够恢复秘密，但不少于$k$玩家的集合可以。这是通过随机和独立地选择$n+k-1$元素$u_0, \ldots, u_{n-1}, f_1, \ldots, f_{k-1} \in \mathbb{F}p$并将$f\left(u_i\right)$提供给玩家$i$来实现的，其中$f=f{k-1} x^{k-1}+$$\cdots+f_1 x+f_0 \in \mathbb{F}_p[x]$和$f_0 \in \mathbb{F}_p$是上述的秘密。同样，需要$u_i \neq u_j$如果$i \neq j$。由于$f$是由其在$k$点上的值唯一确定的，因此$n$玩家中的每个$k$子集都可以计算$f$，从而获得秘密$f_0$，但少于$k$的玩家没有关于$f_0$的信息。

数学代写|现代代数代写Modern Algebra代考|The Chinese Remainder Algorithm

假设$f \in \mathbb{N}$有两个十进制数，除11余数为2，除13余数为7。这是否唯一地定义了$f$，如果是，是否有比检查0到99之间的所有值更好的方法来找到它?在本节中，我们将看到两个问题的答案都是肯定的。
对于本节，$R$是欧几里得域，我们修复以下符号:
$m_0, \ldots, m_{r-1} \in R$是成对的素数，所以$\operatorname{gcd}\left(m_i, m_j\right)=1$
请访问$0 \leq i<j<r$和$m=m_0 \cdots m_{r-1}$。
因此$m=\operatorname{lcm}\left(m_0, \ldots, m_{r-1}\right)$。对于$0 \leq i<r$，我们有正则环同态
$$
\begin{aligned}
\pi_i: R & \longrightarrow R /\left\langle m_i\right\rangle, \
f & \longmapsto f \bmod m_i .
\end{aligned}
$$
把所有这些结合起来$i$，我们得到环同态
$$
\begin{aligned}
& \chi=\pi_0 \times \cdots \times \pi_{r-1}: R \longrightarrow R /\left\langle m_0\right\rangle \times \ldots \times R /\left\langle m_{r-1}\right\rangle, \
& f \longmapsto\left(f \bmod m_0, \ldots, f \bmod m_{r-1}\right) \text {. } \
&
\end{aligned}
$$
对于上面的例子，我们有$R=\mathbb{Z}, r=2, m_0=11, m_1=13, m=143$和
$$
\chi(f)=(f \bmod 11, f \bmod 13)=(2 \bmod 11,7 \bmod 13) \in \mathbb{Z}{11} \times \mathbb{Z}{13} .
$$
下面的语句用有些抽象的术语为我们的许多算法提供了理论基础。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

有限元方法代写

随机分析代写

时间序列分析代写

回归分析代写

MATLAB代写

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Modular inverses via Euclid

Posted on 2023年6月16日2023年6月16日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Modular inverses via Euclid

We have seen in the previous section how modular addition and multiplication works. What about inversion and division? Do expressions like $a^{-1} \bmod m$ and $a / b \bmod m$ make sense, and if so, how can we compute their value? The following theorem gives an answer when the underlying ring $R$ is a Euclidean domain.
THEOREM 4.1.
Let $R$ be a Euclidean domain, $a, m \in R$, and $S=R / m R$. Then $a \bmod m \in S$ is a unit if and only if $\operatorname{gcd}(a, m)=1$. In this case, the modular inverse of $a \bmod m$ can be computed by means of the Extended Euclidean Algorithm.
PROOF. We have
$$
\begin{gathered}
a \text { is invertible modulo } m \Longleftrightarrow \exists s \in R \quad s a \equiv 1 \bmod m \
\quad \Longleftrightarrow \exists s, t \in R \quad s a+t m=1 \Longrightarrow \operatorname{gcd}(a, m)=1 .
\end{gathered}
$$
If, on the other hand, $\operatorname{gcd}(a, m)=1$, then the Extended Euclidean Algorithm provides such $s, t \in R$.

EXAmple 4.2. We let $R=\mathbb{Z}, m=29$, and $a=12$. Then $\operatorname{gcd}(a, m)=1$, and the Extended Euclidean Algorithm computes $5 \cdot 29+(-12) \cdot 12=1$. Thus $(-12) \cdot 12 \equiv$ $17 \cdot 12 \equiv 1 \bmod 29$, and hence 17 is the inverse of 12 modulo 29 .

EXAmple 4.3. Let $R=\mathbb{Q}[x], m=x^3-x+2$, and $a=x^2$. The last row in the Extended Euclidean Algorithm for $m$ and $a$ is
$$
\left(\frac{1}{4} x+\frac{1}{2}\right)\left(x^3-x+2\right)+\left(-\frac{1}{4} x^2-\frac{1}{2} x+\frac{1}{4}\right) x^2=1,
$$
and $\left(-x^2-2 x+1\right) / 4$ is the inverse of $x^2$ modulo $x^3-x+2$.

数学代写|现代代数代写Modern Algebra代考|Repeated squaring

An important tool for modular exponentiation is repeated squaring (or square and multiply). In fact, this technique works in any set with an associative multiplication, but we will mainly use it in residue class rings.
AlGORITHM 4.8 Repeated squaring.
Input: $a \in R$, where $R$ is a ring with 1 , and $n \in \mathbb{N}_{>0}$.
Output: $a^n \in R$.

${$ binary representation of $n}$
write $n=2^k+n_{k-1} \cdot 2^{k-1}+\cdots+n_1 \cdot 2+n_0$, with all $n_i \in{0,1}$
$$
b_k \longleftarrow a
$$

for $i=k-1, k-2, \ldots, 0$ do
$$
\text { if } n_i=1 \text { then } b_i \longleftarrow b_{i+1}^2 a \text { else } b_i \longleftarrow b_{i+1}^2
$$

return $b_0$
Correctness follows easily from the invariant $b_i=a^{\left\lfloor n / 2^i\right\rfloor}$. This procedure uses $\lfloor\log n\rfloor$ squarings plus $w(n)-1 \leq\lfloor\log n\rfloor$ multiplications in $R$, where log is the binary logarithm and $w(n)$ is the Hamming weight of the binary representation of $n$ (Chapter 7), that is, the number of ones in it. Thus the total cost is at most $2 \log n$ multiplications. For example, the binary representation of 13 is $1 \cdot 2^3+$ $1 \cdot 2^2+0 \cdot 2+1$ and has Hamming weight 3 . Thus $a^{13}$ would be computed as $\left(\left(a^2 \cdot a\right)^2\right)^2 \cdot a$, using three squarings and two multiplications. If $R=\mathbb{Z}_{17}=\mathbb{Z} /\langle 17\rangle$ and $a=8 \bmod 17$, then we compute $8^{13} \bmod 17$ as
$$
\begin{aligned}
8^{13} & \equiv\left(\left(8^2 \cdot 8\right)^2\right)^2 \cdot 8 \equiv\left((-4 \cdot 8)^2\right)^2 \cdot 8 \
& \equiv\left(2^2\right)^2 \cdot 8=4^2 \cdot 8 \equiv-1 \cdot 8=-8 \bmod 17
\end{aligned}
$$
which is much faster than first evaluating $8^{13}=549755813888$ and then dividing by 17 with remainder. This method was already used by Euler (1761). He calculated $7^{160} \bmod 641$ by computing $7^2, 7^4, 7^8, 7^{16}, 7^{32}, 7^{64}, 7^{128}, 7^{160}=7^{128} \cdot 7^{32}$, reducing modulo 641 after each step. (He also listed, unnecessarily, $7^3$.) As another example, starting from $2^{2^3}=2^8=256$, we only need two squarings modulo $5 \cdot 2^7+1=641$ to calculate $\left(\left(2^8\right)^2\right)^2=2^{2^5} \equiv-1 \bmod 641$. This shows that 641 divides the fifth Fermat number $F_5=2^{2^5}+1$, as discovered by Euler (1732/33); see Sections 18.2 and 19.1. Even if we were given the 10 -digit number $2^{2^5}+1=$ 4294967297 , it would seem more laborious to divide it by 641 with remainder rather than to use modular repeated squaring.

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Modular inverses via Euclid

在前一节中，我们已经看到了模块化加法和乘法是如何工作的。反转和除法呢?像$a^{-1} \bmod m$和$a / b \bmod m$这样的表达式有意义吗?如果有意义，我们如何计算它们的值?下面的定理给出了当下面的环$R$是欧几里得域时的答案。
定理4.1。
设$R$为欧几里得域，$a, m \in R$和$S=R / m R$。那么$a \bmod m \in S$是一个单位当且仅当$\operatorname{gcd}(a, m)=1$。在这种情况下，可以通过扩展欧几里得算法计算$a \bmod m$的模逆。
证明。我们有
$$
\begin{gathered}
a \text { is invertible modulo } m \Longleftrightarrow \exists s \in R \quad s a \equiv 1 \bmod m \
\quad \Longleftrightarrow \exists s, t \in R \quad s a+t m=1 \Longrightarrow \operatorname{gcd}(a, m)=1 .
\end{gathered}
$$
另一方面，如果$\operatorname{gcd}(a, m)=1$，那么扩展欧几里得算法提供了这样的$s, t \in R$。

例4.2。我们让$R=\mathbb{Z}, m=29$和$a=12$。然后$\operatorname{gcd}(a, m)=1$，扩展欧几里得算法计算$5 \cdot 29+(-12) \cdot 12=1$。因此$(-12) \cdot 12 \equiv$$17 \cdot 12 \equiv 1 \bmod 29$，因此17是12模29的倒数。

例4.3。设$R=\mathbb{Q}[x], m=x^3-x+2$和$a=x^2$。扩展欧几里得算法中$m$和$a$的最后一行是
$$
\left(\frac{1}{4} x+\frac{1}{2}\right)\left(x^3-x+2\right)+\left(-\frac{1}{4} x^2-\frac{1}{2} x+\frac{1}{4}\right) x^2=1,
$$
$\left(-x^2-2 x+1\right) / 4$是$x^2$模$x^3-x+2$的倒数。

数学代写|现代代数代写Modern Algebra代考|Repeated squaring

模求幂的一个重要工具是重复平方(或平方乘以)。实际上，这种技术适用于任何具有关联乘法的集合，但我们主要在剩余类环中使用它。
4.8重复平方。
输入:$a \in R$，其中$R$是一个带1的环，$n \in \mathbb{N}_{>0}$。
输出:$a^n \in R$。

${$$n}$的二进制表示
写$n=2^k+n_{k-1} \cdot 2^{k-1}+\cdots+n_1 \cdot 2+n_0$，用所有 $n_i \in{0,1}$
$$
b_k \longleftarrow a
$$

对于$i=k-1, k-2, \ldots, 0$ do
$$
\text { if } n_i=1 \text { then } b_i \longleftarrow b_{i+1}^2 a \text { else } b_i \longleftarrow b_{i+1}^2
$$

返回$b_0$
正确性很容易从不变量$b_i=a^{\left\lfloor n / 2^i\right\rfloor}$得到。这个过程在$R$中使用$\lfloor\log n\rfloor$平方加上$w(n)-1 \leq\lfloor\log n\rfloor$乘法，其中log是二进制对数，$w(n)$是$n$二进制表示的汉明权重(第七章)，即其中的1的个数。因此，总成本最多为$2 \log n$次乘法。例如，13的二进制表示为$1 \cdot 2^3+$$1 \cdot 2^2+0 \cdot 2+1$，其汉明权值为3。因此，使用三次平方和两次乘法，$a^{13}$将被计算为$\left(\left(a^2 \cdot a\right)^2\right)^2 \cdot a$。如果$R=\mathbb{Z}_{17}=\mathbb{Z} /\langle 17\rangle$和$a=8 \bmod 17$，那么我们计算$8^{13} \bmod 17$等于
$$
\begin{aligned}
8^{13} & \equiv\left(\left(8^2 \cdot 8\right)^2\right)^2 \cdot 8 \equiv\left((-4 \cdot 8)^2\right)^2 \cdot 8 \
& \equiv\left(2^2\right)^2 \cdot 8=4^2 \cdot 8 \equiv-1 \cdot 8=-8 \bmod 17
\end{aligned}
$$
这比先求$8^{13}=549755813888$然后除以17余数要快得多。欧拉(1761)已经使用了这种方法。他通过计算$7^2, 7^4, 7^8, 7^{16}, 7^{32}, 7^{64}, 7^{128}, 7^{160}=7^{128} \cdot 7^{32}$来计算$7^{160} \bmod 641$，每一步减少模数641。(他还不必要地列出了$7^3$。)作为另一个例子，从$2^{2^3}=2^8=256$开始，我们只需要两次对$5 \cdot 2^7+1=641$取模来计算$\left(\left(2^8\right)^2\right)^2=2^{2^5} \equiv-1 \bmod 641$。这表明欧拉(1732/33)发现的第五个费马数$F_5=2^{2^5}+1$可以被641整除;参见18.2节和19.1节。即使我们得到10位数$2^{2^5}+1=$ 4294967297，用它除以带余数的641似乎比使用模重复平方更费力。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

有限元方法代写

随机分析代写

时间序列分析代写

回归分析代写

MATLAB代写

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|Representation and addition of numbers

Posted on 2023年6月16日2023年6月16日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|Representation and addition of numbers

Algebra starts with numbers and computers work on data, so the very first issue in computer algebra is how to feed numbers as data into computers. Data are stored in pieces called words. Current machines use either 32- or 64-bit words; to be specific, we assume that we have a 64-bit processor. Then one machine word contains a single precision integer between 0 and $2^{64}-1$.

How can we represent integers outside the range $\left{0, \ldots, 2^{64}-1\right}$ ? Such a multiprecision integer is represented by an array of 64-bit words, where the first one encodes the sign of the integer and the length of the array. To be precise, we consider the $2^{64}$-ary (or radix $2^{64}$ ) representation of a nonzero integer
$$
a=(-1)^s \sum_{0 \leq i \leq n} a_i \cdot 2^{64 i}
$$
where $s \in{0,1}, 0 \leq n+1<2^{63}$, and $a_i \in\left{0, \ldots, 2^{64}-1\right}$ for all $i$ are the digits (in base $2^{64}$ ) of $a$. We encode it as an array
$$
s \cdot 2^{63}+n+1, a_0, \ldots, a_n
$$
of 64-bit words. This representation can be made unique by requiring that the leading digit $a_n$ be nonzero if $a \neq 0$ (and using the single-entry array 0 to represent $a=0$ ). We will call this the standard representation for $a$. For example, the standard representation of -1 is $2^{63}+1,1$. It is, however, convenient also to allow nonstandard representations with leading zero digits since this sometimes facilitates memory management, but we do not want to go into details here. The range of integers that can be represented in standard representation on a 64-bit processor is between $-2^{64 \cdot 2^{63}}+1$ and $2^{64 \cdot 2^{63}}-1$; each of the two boundaries requires $2^{63}+1$ words of storage. This size limitation is quite sufficient for practical purposes: one of the larger representable numbers would fill about 70 million 1-TB-discs.
For a nonzero integer $a \in \mathbb{Z}$, we define the length $\lambda(a)$ of $a$ as
$$
\lambda(a)=\left\lfloor\log _{2^{64}}|a|\right\rfloor+1=\left\lfloor\frac{\log _2|a|}{64}\right\rfloor+1,
$$
where $\lfloor\cdot\rfloor$ denotes rounding down to the nearest integer (so that $\lfloor 2.7\rfloor=2$ and $\lfloor-2.7\rfloor=-3$ ). Thus $\lambda(a)+1=n+2$ is the number of words in the standard representation (1) of $a$ (see Exercise 2.1). This is quite a cluttered expression, and it is usually sufficient to know that about $\frac{1}{64} \log _2|a|$ words are needed, or even more succinctly $O\left(\log _2|a|\right)$, where the big-Oh notation ” $O$ ” hides an arbitrary constant (Section 25.7).

数学代写|现代代数代写Modern Algebra代考|Representation and addition of polynomials

The two main data types on which our algorithms operate are numbers as above and polynomials, such as $a=5 x^3-4 x+3 \in \mathbb{Z}[x]$. In general, we have a commutative ring $R$, such as $\mathbb{Z}$, in which we can perform the operations of addition, subtraction, and multiplication according to the usual rules; see Section 25.2 for details. (All our rings have a multiplicative unit element 1.) If we can also divide by any nonzero element, as in the rational numbers $\mathbb{Q}$, then $R$ is a field.

A polynomial $a \in R[x]$ in $x$ over $R$ is a finite sequence $\left(a_0, \ldots, a_n\right)$ of elements of $R$ (the coefficients of $a$ ), for some $n \in \mathbb{N}$, and we write it as
$$
a=a_n x^n+a_{n-1} x^{n-1}+\cdots+a_1 x+a_0=\sum_{0 \leq i \leq n} a_i x^i .
$$
If $a_n \neq 0$, then $n=\operatorname{deg} a$ is the degree of $a$, and $a_n=\operatorname{lc}(a)$ is its leading coefficient. If $\operatorname{lc}(a)=1$, then $a$ is monic. It is convenient to take $-\infty$ as the degree of the zero polynomial. We can represent $a$ by an array whose $i$ th element is $a_i$ (in analogy to the integer case, we would also need some storage for the degree, but we will neglect this). This assumes that we already have a way of representing coefficients from $R$. The length (the number of ring elements) of this representation is $n+1$.
For an integer $r \in \mathbb{N}{>1}$ (in particular, for $r=2^{64}$ as in the previous section), the representations (2) of a polynomial and the radix $r$ representation $$ a=a_n r^n+a{n-1} r^{n-1}+\cdots+a_1 r+a_0=\sum_{0 \leq i \leq n} a_i r^i,
$$
with digits $a_0, \ldots, a_n \in{0, \ldots, r-1}$, of an integer $a$ are quite similar. This is particularly visible if we take polynomials over $R=\mathbb{Z}r={0, \ldots, r-1}$, the ring of integers modulo $r$, with addition and multiplication modulo $r$ (Sections 4.1 and 25.2). This similarity is an important point for computer algebra; many of our algorithms apply (with small modifications) to both the integer and polynomial cases: multiplication, division with remainder, ged and Chinese remainder computation. It is also relevant to note where this does not apply: the subresultant theory (Chapter 6) and, most importantly, the factorization problem (Parts III and IV). At the heart of this distinction lies the deceptively simple carry rule. It gives the low digits some influence on the high digits in addition of integers, and messes up the cleanly separated rules in the addition of two polynomials $$ a=\sum{0 \leq i \leq n} a_i x^i \text { and } b=\sum_{0 \leq i \leq m} b_i x^i
$$
in $R[x]$. This is quite easy:
$$
c=a+b=\sum_{0 \leq i \leq n}\left(a_i+b_i\right) x^i=\sum_{0 \leq i \leq n} c_i x^i,
$$
where the addition $c_i=a_i+b_i$ is performed in $R$ and, as with integers, we may assume that $m=n$.

现代代数代考

数学代写|现代代数代写Modern Algebra代考|Representation and addition of numbers

代数从数字开始，计算机处理数据，所以计算机代数的第一个问题是如何将数字作为数据输入计算机。数据以块的形式存储，称为字。当前的机器使用32位或64位字;具体地说，我们假设我们有一个64位处理器。然后一个机器字包含0到$2^{64}-1$之间的单个精度整数。

如何表示$\left{0, \ldots, 2^{64}-1\right}$范围之外的整数?这样的多精度整数由64位单词数组表示，其中第一个单词编码整数的符号和数组的长度。准确地说，我们考虑一个非零整数的$2^{64}$ -任意(或基数$2^{64}$)表示
$$
a=(-1)^s \sum_{0 \leq i \leq n} a_i \cdot 2^{64 i}
$$
其中$s \in{0,1}, 0 \leq n+1<2^{63}$和$a_i \in\left{0, \ldots, 2^{64}-1\right}$对于所有的$i$都是$a$的数字(以$2^{64}$为基数)。我们将其编码为数组
$$
s \cdot 2^{63}+n+1, a_0, \ldots, a_n
$$
64位字。通过要求$a \neq 0$的前导数字$a_n$非零(并使用单条目数组0来表示$a=0$)，可以使这种表示惟一。我们将其称为$a$的标准表示。例如，-1的标准表示形式是$2^{63}+1,1$。然而，允许前导数字为零的非标准表示也很方便，因为这有时有助于内存管理，但我们不想在这里详细讨论。在64位处理器上可以用标准表示法表示的整数范围在$-2^{64 \cdot 2^{63}}+1$和$2^{64 \cdot 2^{63}}-1$之间;这两个边界中的每一个都需要$2^{63}+1$字的存储。这个大小限制对于实际目的来说是足够的:一个较大的可表示数字将填充大约7000万个1 tb的磁盘。
对于非零整数$a \in \mathbb{Z}$，我们定义$a$的长度$\lambda(a)$为
$$
\lambda(a)=\left\lfloor\log _{2^{64}}|a|\right\rfloor+1=\left\lfloor\frac{\log _2|a|}{64}\right\rfloor+1,
$$
其中$\lfloor\cdot\rfloor$表示舍入到最接近的整数(因此$\lfloor 2.7\rfloor=2$和$\lfloor-2.7\rfloor=-3$)。因此$\lambda(a)+1=n+2$是$a$(参见练习2.1)的标准表示(1)中的字数。这是一个相当混乱的表达式，通常知道需要大约$\frac{1}{64} \log _2|a|$个单词就足够了，或者更简洁地说$O\left(\log _2|a|\right)$，其中大哦符号“$O$”隐藏了一个任意常数(第25.7节)。

数学代写|现代代数代写Modern Algebra代考|Representation and addition of polynomials

我们的算法操作的两种主要数据类型是上面提到的数字和多项式，比如$a=5 x^3-4 x+3 \in \mathbb{Z}[x]$。一般来说，我们有一个交换环$R$，比如$\mathbb{Z}$，在这个交换环中，我们可以按照通常的规则进行加、减、乘的运算;详细信息请参见第25.2节。(所有的环都有一个乘法单位元素1。)如果我们也可以除以任何非零元素，如有理数$\mathbb{Q}$，那么$R$是一个字段。

在$x$ / $R$中的多项式$a \in R[x]$是$R$元素($a$的系数)的有限序列$\left(a_0, \ldots, a_n\right)$，对于某些$n \in \mathbb{N}$，我们把它写成
$$
a=a_n x^n+a_{n-1} x^{n-1}+\cdots+a_1 x+a_0=\sum_{0 \leq i \leq n} a_i x^i .
$$
若为$a_n \neq 0$，则$n=\operatorname{deg} a$为$a$的度，$a_n=\operatorname{lc}(a)$为其超前系数。如果是$\operatorname{lc}(a)=1$，那么$a$就是monic。用$-\infty$作为零多项式的阶是方便的。我们可以用一个数组来表示$a$，其$i$元素为$a_i$(与整数情况类似，我们也需要一些度数存储空间，但我们将忽略这一点)。这里假设我们已经有了一种表示$R$中系数的方法。这个表示的长度(环元素的数量)是$n+1$。
对于整数$r \in \mathbb{N}{>1}$(特别是上一节中的$r=2^{64}$)，多项式的表示(2)和基数的表示$r$$$ a=a_n r^n+a{n-1} r^{n-1}+\cdots+a_1 r+a_0=\sum_{0 \leq i \leq n} a_i r^i,
$$
对于数字$a_0, \ldots, a_n \in{0, \ldots, r-1}$，整数$a$是非常相似的。如果我们在$R=\mathbb{Z}r={0, \ldots, r-1}$上取多项式，这是特别明显的，整数环以$r$为模，加法和乘法以$r$为模(第4.1和25.2节)。这种相似性对于计算机代数来说是很重要的一点;我们的许多算法(稍加修改)既适用于整数情况，也适用于多项式情况:乘法、余数除法、格数和中国余数计算。同样值得注意的是，这并不适用于子结果理论(第6章)和最重要的因式分解问题(第三和第四部分)。这种区别的核心在于看似简单的进位规则。在整数相加的过程中，低位数对高位数有一定的影响，并且在两个多项式相加的过程中混淆了清晰分离的规则$$ a=\sum{0 \leq i \leq n} a_i x^i \text { and } b=\sum_{0 \leq i \leq m} b_i x^i
$$
在$R[x]$。这很简单:
$$
c=a+b=\sum_{0 \leq i \leq n}\left(a_i+b_i\right) x^i=\sum_{0 \leq i \leq n} c_i x^i,
$$
在$R$中执行加法$c_i=a_i+b_i$，与整数一样，我们可以假设$m=n$。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

有限元方法代写

随机分析代写

时间序列分析代写

回归分析代写

MATLAB代写

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|BOOLEAN ALGEBRAS

Posted on 2023年6月16日2023年6月16日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|BOOLEAN ALGEBRAS

In 1854 the British mathematician George Boole published a book entitled An Investigation of the Laws of Thought, on Which Are Founded the Mathematical Theories of Logic and Probabilities. This book amplified ideas Boole had introduced in a shorter work published in 1847 , and brought the study of logic clearly into the domain of mathematics. Boolean algebra, which originated with this work, can now be seen as the proper tool for the study not only of algebraic logic, but also such things as the theory of telephone switching circuits and computer design.

Definition I. A Boolean algebra is a lattice with zero (0) and unity (1) that is distributive and complemented.

Lattices were presented in two forms in Section 64: first in the definition, in terms of a partial ordering $\leq$, and then in Theorem 64.1 , in terms of two operations $\vee$ and $\wedge$. Boolean algebras are most often discussed in the second of these forms. Because of this we next give an alternative to Definition I. Theorem 65.1 establishes the equivalence of the two definitions. Hereafter, you may work only from Definition II, if you like. All that is required from Theorem 65.1 is the definition of $\leq$ given in (65.1), and the fact that this gives a partial ordering in a Boolean algebra as defined in Definition II.

Definition II. A Boolean algebra is a set $B$ together with two operations $\vee$ and $\wedge$ on $B$ such that each of the following axioms is satisfied (for all $a, b, c \in B$ ):
Commutative laws
$$
a \vee b=b \vee a, \quad a \wedge b=b \wedge a
$$
Associative laws
$$
a \vee(b \vee c)=(a \vee b) \vee c, \quad a \wedge(b \wedge c)=(a \wedge b) \wedge c,
$$
Distributive laws
$$
a \wedge(b \vee c)=(a \wedge b) \vee(a \wedge c), \quad a \vee(b \wedge c)=(a \vee b) \wedge(a \vee c),
$$
Existence of zero and unity
There are elements 0 and 1 in $B$ such that
$$
a \vee 0=a, \quad a \wedge 1=a,
$$
Existence of complements
For each $a$ in $B$ there is an element $a^{\prime}$ in $B$ such that
$$
a \vee a^{\prime}=1 \text { and } a \wedge a^{\prime}=0 .
$$

数学代写|现代代数代写Modern Algebra代考|FINITE BOOLEAN ALGEBRAS

The goal of this section is to prove Theorem 66.1 , which characterizes all finite Boolean algebras. Boolean algebras, like groups and other algebraic structures, are classified according to isomorphism.

Definition. If $A$ and $B$ are Boolean algebras, an isomorphism of $A$ onto $B$ is a mapping $\theta: A \rightarrow B$ that is one-to-one and onto and satisfies
$$
\theta(a \vee b)=\theta(a) \vee \theta(b)
$$
and
$$
\theta(a \wedge b)=\theta(a) \wedge \theta(b)
$$
for all $a, b \in A$. If there is an isomorphism of $A$ onto $B$, then $A$ and $B$ are said to be isomorphic, and we write $A \approx B$.

Theorem 66.1. Every finite Boolean algebra is isomorphic to the Boolean algebra of all subsets of some finite set.

Example 66.1. The divisors of 30 form a Boolean algebra with $a \leq b$ defined to mean $a \mid b$. Its diagram is shown in Figure 66.1. Here $a \vee b$ is the least common multiple of $a$ and $b$, and $a \wedge b$ is the greatest common divisor of $a$ and $b$.

A comparison of Figure 66.1 with Figure 63.1 , the diagram for the Boolean algebra of subsets of ${x, y, z}$, suggests an isomorphism determined by $\theta(2)={x}$, $\theta(3)={y}$, and $\theta(5)={z}$. The condition $\theta(a \vee b)=\theta(a) \vee \theta(b)$ forces $\theta(6)={x, y}$, $\theta(10)={x, z}, \theta(15)={y, z}$, and $\theta(30)={x, y, z}$. Also, the condition $\theta(a \wedge b)=$ $\theta(a) \wedge \theta(b)$ forces $\theta(1)=\emptyset$. This mapping $\theta$ is an isomorphism. The idea here is to match the elements covering 1 (the prime divisors of 30 ) with the elements covering $\emptyset$ (the singleelement subsets of ${x, y, z})$. This simple and important idea is the key to Theorem 66.1.
(Although the divisors of 30 form a Boolean algebra relative to $a \mid b$, the divisors of 12 (Figure 63.2) do not, because 6 has no complement among the divisors of 12 . See Problem 66.7 for a more general statement.)

现代代数代考

数学代写|现代代数代写Modern Algebra代考|BOOLEAN ALGEBRAS

1854年，英国数学家乔治·布尔出版了一本名为《思维规律的研究》的书，《思维规律是逻辑和概率论的数学理论的基础》。这本书扩大了布尔在1847年发表的一篇较短的著作中介绍的思想，并将逻辑研究清楚地带入了数学领域。布尔代数起源于这项工作，现在不仅可以被视为研究代数逻辑的合适工具，而且还可以被视为研究电话交换电路理论和计算机设计的合适工具。

定义一:布尔代数是具有零(0)和单位(1)的分配型补格。

在第64节中，格以两种形式呈现:首先在定义中，以偏序$\leq$的形式呈现，然后在定理64.1中，以两个操作$\vee$和$\wedge$的形式呈现。布尔代数通常以第二种形式讨论。因此，我们接下来给出定义一的另一种选择。定理65.1确立了这两个定义的等价性。以后，如果你愿意，你可以只从定义II开始工作。定理65.1所需要的就是(65.1)中给出的$\leq$的定义，以及它给出了定义II中定义的布尔代数中的偏序。

定义二。布尔代数是一个集合$B$以及$B$上的两个操作$\vee$和$\wedge$，使得以下公理满足(对于所有$a, b, c \in B$):
交换律
$$
a \vee b=b \vee a, \quad a \wedge b=b \wedge a
$$
结合律
$$
a \vee(b \vee c)=(a \vee b) \vee c, \quad a \wedge(b \wedge c)=(a \wedge b) \wedge c,
$$
分配律
$$
a \wedge(b \vee c)=(a \wedge b) \vee(a \wedge c), \quad a \vee(b \wedge c)=(a \vee b) \wedge(a \vee c),
$$
零的存在性和统一性
$B$中的元素0和1使得
$$
a \vee 0=a, \quad a \wedge 1=a,
$$
补语的存在性
对于$B$中的每个$a$, $B$中都有一个元素$a^{\prime}$
$$
a \vee a^{\prime}=1 \text { and } a \wedge a^{\prime}=0 .
$$

数学代写|现代代数代写Modern Algebra代考|FINITE BOOLEAN ALGEBRAS

本节的目的是证明定理66.1，它是所有有限布尔代数的特征。布尔代数，像群和其他代数结构一样，是根据同构进行分类的。

定义。如果$A$和$B$是布尔代数，那么$A$到$B$的同构是一个映射$\theta: A \rightarrow B$，它是一对一的，并且满足
$$
\theta(a \vee b)=\theta(a) \vee \theta(b)
$$
和
$$
\theta(a \wedge b)=\theta(a) \wedge \theta(b)
$$
对于所有$a, b \in A$。如果$A$和$B$是同构的，那么$A$和$B$就是同构的，我们写$A \approx B$。

定理66.1。每一个有限布尔代数都与某有限集合的所有子集的布尔代数同构。

例66.1。30的因数构成一个布尔代数，其中$a \leq b$定义为$a \mid b$。其示意图如图66.1所示。这里$a \vee b$是$a$和$b$的最小公倍数，$a \wedge b$是$a$和$b$的最大公约数。

将图66.1与图63.1 (${x, y, z}$子集的布尔代数图)进行比较，可以发现由$\theta(2)={x}$、$\theta(3)={y}$和$\theta(5)={z}$确定的同构关系。条件$\theta(a \vee b)=\theta(a) \vee \theta(b)$强制使用$\theta(6)={x, y}$、$\theta(10)={x, z}, \theta(15)={y, z}$和$\theta(30)={x, y, z}$。此外，条件$\theta(a \wedge b)=$$\theta(a) \wedge \theta(b)$迫使$\theta(1)=\emptyset$。这个映射$\theta$是一个同构。这里的想法是将覆盖1(30的质因数)的元素与覆盖$\emptyset$ (${x, y, z})$的单元素子集)的元素进行匹配。这个简单而重要的概念是定理66.1的关键。
(虽然30的因数相对于$a \mid b$形成了一个布尔代数，但12的因数(图63.2)却不是，因为6在12的因数中没有补数。参见问题66.7得到更一般的说明。)

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

金融工程代写

非参数统计代写

非参数统计指的是一种统计方法，其中不假设数据来自于由少数参数决定的规定模型；这种模型的例子包括正态分布模型和线性回归模型。

广义线性模型代考

广义线性模型（GLM）归属统计学领域，是一种应用灵活的线性回归模型。该模型允许因变量的偏差分布有除了正态分布之外的其它分布。

有限元方法代写

随机分析代写

时间序列分析代写

回归分析代写

MATLAB代写

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写

数学代写|现代代数代写Modern Algebra代考|GROUPS ACTING ON SETS

Posted on 2023年6月2日2023年6月2日 by statistics-lab

数学代写|现代代数代写Modern Algebra代考|GROUPS ACTING ON SETS

We begin by recalling the group of symmetries of a square, from Example 8.1. For convenience, here are the definitions of the elements and also the accompanying figure, Figure 56.1.

Group of symmetries of a square (Figure 56.1)
$$
\begin{aligned}
\mu_0 & =\text { identity permutation } \
\mu_{90} & =\text { rotation } 90^{\circ} \text { clockwise around } p \
\mu_{180} & =\text { rotation } 180^{\circ} \text { clockwise around } p \
\mu_{270} & =\text { rotation } 270^{\circ} \text { clockwise around } p \
\rho_H & =\text { reflection through } H \
\rho_V & =\text { reflection through } V \
\rho_1 & =\text { reflection through } D_1 \
\rho_2 & =\text { reflection through } D_2 .
\end{aligned}
$$
Denote the group by $G$. The elements of $G$ are isometries of the plane; therefore, they are permutations of the set of all points of the plane. But these isometries also induce permutations of the set of vertices of the square in a natural way. If we assign to eachisometry the corresponding permutation of ${a, b, c, d}$, then we have a mapping from $G$ to $\operatorname{Sym}{a, b, c, d}$. The mapping with the induced permutations written in cycle notation is
$$
\begin{aligned}
& \mu_0 \mapsto(a)(b)(c)(d) \quad \rho_H \mapsto(a d)(b \quad c) \
& \mu_{90} \mapsto\left(\begin{array}{llll}
a & b & c & d
\end{array}\right) \quad \rho_V \mapsto\left(\begin{array}{lll}
a & b
\end{array}\right)\left(\begin{array}{ll}
c & d
\end{array}\right) \
& \mu_{180} \mapsto(a c)(b d) \quad \rho_1 \mapsto(a)(c)(b d) \
& \mu_{270} \mapsto(a d c b) \quad \rho_2 \mapsto(a c)(b)(d) \text {. } \
&
\end{aligned}
$$
Because the operations on both $G$ and $\operatorname{Sym}{a, b, c, d}$ are composition, this mapping is a homomorphism.

The isometries in $G$ also induce permutations of the set of diagonals $\left{D_1, D_2\right}$ in a natural way. For instance, $\mu_{180}\left(D_1\right)=D_1$ and $\mu_{180}\left(D_2\right)=D_2$ ( $\mu_{180}$ interchanges the ends of each of the diagonals, but that is not important here). In this case we can assign to each isometry the corresponding permutation of $\left{D_1, D_2\right}$. This gives a mapping from $G$ to $\operatorname{Sym}\left{D_1, D_2\right}$. The induced permutations are
$$
\begin{aligned}
& \mu_0 \mapsto\left(D_1\right)\left(D_2\right) \quad \rho_H \mapsto\left(\begin{array}{ll}
D_1 & D_2
\end{array}\right) \
& \mu_{90} \quad \mapsto\left(\begin{array}{lll}
D_1 & D_2
\end{array}\right) \quad \rho_V \quad \mapsto\left(\begin{array}{ll}
D_1 & D_2
\end{array}\right) \
& \mu_{180} \mapsto\left(D_1\right)\left(D_2\right) \quad \rho_1 \quad \mapsto\left(D_1\right)\left(D_2\right) \
& \mu_{270} \mapsto\left(\begin{array}{lll}
D_1 & D_2
\end{array}\right) \quad \rho_2 \mapsto\left(D_1\right)\left(D_2\right) \text {. } \
&
\end{aligned}
$$

数学代写|现代代数代写Modern Algebra代考|BURNSIDE’S COUNTING THEOREM

One of the central problems of combinatorics is to compute the number of distinguishable ways in which something can be done. A simple example is to compute the number of distinguishable ways the three edges of an equilateral triangle can be painted so that one edge is red $(R)$, one is white $(W)$, and one is blue $(B)$. The six possibilities are shown in the top row of Figure 57.1.

If we permit rotation of the triangle in the plane, then the first three possibilities become indistinguishable-they collapse into the first possibility in the second row of Figure 57.1. The last three possibilities in the top row collapse into the second possibility in the second row.

If we permit reflections through lines, as well as rotations in the plane, then the only possibility is that shown in the third row of Figure 57.1.

In passing from one row to the next in the example, we have treated different ways of painting the triangle as being equivalent, and we have shown one representative from each equivalence class. The problem at each step is to compute the number of equivalence classes. In the terminology used most often in combinatorics, possibilities in the same equivalence class are indistinguishable, whereas possibilities in different equivalence classes are distinguishable. So the problem of computing the number of distinguishable ways in which something can be done is the same as that of computing the number of equivalence classes under an appropriate equivalence relation. The link between group theory and combinatorics rests on the fact that the equivalence classes are very often orbits under the action of an appropriate group. In the example, the appropriate group for passing to the second row of Figure 57.1 is the group of rotations of the triangle; the appropriate group for passing to the third row contains these three rotations and also three reflections, each through a line connecting a vertex with the midpoint of the opposite side (this group is $D_3$ in the notation of Section 59).

To solve a combinatorics problem with these ideas, the first step is to get clearly in mind the total set of possibilities (without regard to equivalence). Next, decide the condition under which possibilities are to be considered equivalent (indistinguishable). This will mean membership in a common orbit under the action of some group; so in effect this means to choose an appropriate group. Finally, compute the number of orbits relative to this group; this will be the number of distinguishable possibilities. This method derives its power from Burnside’s Counting Theorem, proved below, which gives a way to compute the number of orbits relative to a group action (William Burnside, 1852-1927).

现代代数代考

数学代写|现代代数代写Modern Algebra代考|GROUPS ACTING ON SETS

我们首先回顾例8.1中正方形的一组对称性。为方便起见，这里列出了元素的定义以及附带的图，图56.1。

正方形的对称组(图56.1)
$$
\begin{aligned}
\mu_0 & =\text { identity permutation } \
\mu_{90} & =\text { rotation } 90^{\circ} \text { clockwise around } p \
\mu_{180} & =\text { rotation } 180^{\circ} \text { clockwise around } p \
\mu_{270} & =\text { rotation } 270^{\circ} \text { clockwise around } p \
\rho_H & =\text { reflection through } H \
\rho_V & =\text { reflection through } V \
\rho_1 & =\text { reflection through } D_1 \
\rho_2 & =\text { reflection through } D_2 .
\end{aligned}
$$
用$G$表示组。$G$的元素是平面的等距;因此，它们是平面上所有点的集合的置换。但是这些等距也以一种自然的方式引起了正方形顶点集合的排列。如果我们给每个等距分配${a, b, c, d}$对应的排列，那么我们就有了一个从$G$到$\operatorname{Sym}{a, b, c, d}$的映射。用循环表示法表示的与诱导置换的映射是
$$
\begin{aligned}
& \mu_0 \mapsto(a)(b)(c)(d) \quad \rho_H \mapsto(a d)(b \quad c) \
& \mu_{90} \mapsto\left(\begin{array}{llll}
a & b & c & d
\end{array}\right) \quad \rho_V \mapsto\left(\begin{array}{lll}
a & b
\end{array}\right)\left(\begin{array}{ll}
c & d
\end{array}\right) \
& \mu_{180} \mapsto(a c)(b d) \quad \rho_1 \mapsto(a)(c)(b d) \
& \mu_{270} \mapsto(a d c b) \quad \rho_2 \mapsto(a c)(b)(d) \text {. } \
&
\end{aligned}
$$
因为$G$和$\operatorname{Sym}{a, b, c, d}$上的操作都是复合的，所以这个映射是同态的。

$G$中的等距也以自然的方式诱导了一组对角线$\left{D_1, D_2\right}$的排列。例如，$\mu_{180}\left(D_1\right)=D_1$和$\mu_{180}\left(D_2\right)=D_2$ ($\mu_{180}$互换了每个对角线的两端，但这在这里并不重要)。在这种情况下，我们可以为每个等距分配$\left{D_1, D_2\right}$的相应排列。这给出了从$G$到$\operatorname{Sym}\left{D_1, D_2\right}$的映射。诱导排列为
$$
\begin{aligned}
& \mu_0 \mapsto\left(D_1\right)\left(D_2\right) \quad \rho_H \mapsto\left(\begin{array}{ll}
D_1 & D_2
\end{array}\right) \
& \mu_{90} \quad \mapsto\left(\begin{array}{lll}
D_1 & D_2
\end{array}\right) \quad \rho_V \quad \mapsto\left(\begin{array}{ll}
D_1 & D_2
\end{array}\right) \
& \mu_{180} \mapsto\left(D_1\right)\left(D_2\right) \quad \rho_1 \quad \mapsto\left(D_1\right)\left(D_2\right) \
& \mu_{270} \mapsto\left(\begin{array}{lll}
D_1 & D_2
\end{array}\right) \quad \rho_2 \mapsto\left(D_1\right)\left(D_2\right) \text {. } \
&
\end{aligned}
$$

数学代写|现代代数代写Modern Algebra代考|BURNSIDE’S COUNTING THEOREM

组合学的核心问题之一是计算完成某件事的不同方法的数量。一个简单的例子是计算等边三角形的三条边的不同绘制方法的数量，一条边是红色(R)$，一条是白色(W)$，一条是蓝色(B)$。图57.1的最上面一行显示了六种可能性。

如果我们允许在平面上旋转三角形，那么前三种可能性将变得无法区分—它们将合并为图57.1第二行中的第一种可能性。上面一行的最后三种可能会变成第二行的第二种可能。

如果我们允许通过线反射，以及在平面上的旋转，那么唯一的可能性是图57.1的第三行所示。

在示例中，从一行传递到下一行时，我们处理了将三角形画成等价的不同方法，并从每个等价类中展示了一个代表。每一步的问题是计算等价类的数量。在组合学中最常用的术语中，同一等价类中的可能性是不可区分的，而不同等价类中的可能性是可区分的。因此，计算某件事的可区分方法的数目的问题与计算在适当的等价关系下等价类的数目的问题是一样的。群论和组合学之间的联系建立在这样一个事实之上:等价类常常是在适当群的作用下运行的轨道。在本例中，传递到图57.1的第二行的适当组是三角形的旋转组;传递到第三行的合适的组包含这三个旋转和三个反射，每个都通过一条连接顶点和对边中点的线(在第59节的符号中，这个组是$D_3$)。

要用这些想法解决组合问题，第一步是要清楚地记住所有的可能性(不考虑等价性)。接下来，决定在何种条件下可能性被认为是等价的(不可区分的)。这将意味着在某个集团的作用下，在一个共同轨道上的成员;实际上，这意味着选择一个合适的群体。最后，计算相对于该组的轨道数;这是可区分可能性的数目。这种方法的力量来自伯恩赛德的计数定理，下面证明了它，它提供了一种计算相对于群作用的轨道数的方法(威廉·伯恩赛德，1852-1927)。

统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。

R语言代写	问卷设计与分析代写
PYTHON代写	回归分析与线性模型代写
MATLAB代写	方差分析与试验设计代写
STATA代写	机器学习/统计学习代写
SPSS代写	计量经济学代写
EVIEWS代写	时间序列分析代写
EXCEL代写	深度学习代写
SQL代写	各种数据建模与可视化代写