Statistics-lab™可以为您提供ucc.edu МАТ143 Mathematical Analysis数学分析的代写代考和辅导服务！

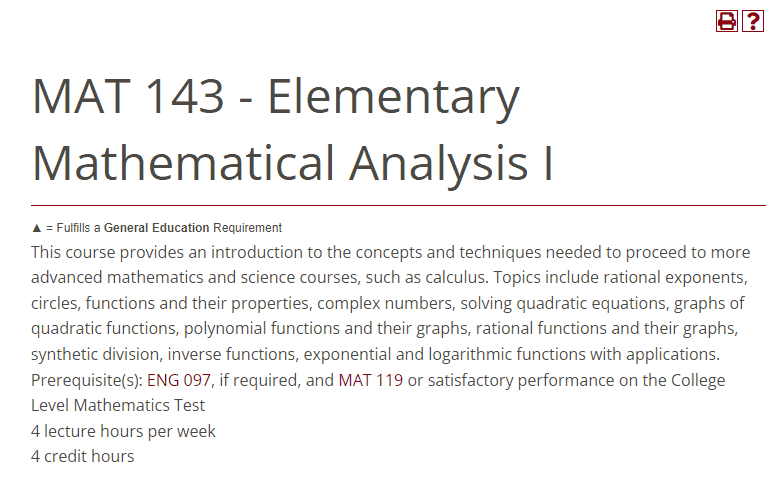
МАТ143 Mathematical Analysis课程简介

Precalculus is a foundational course that prepares students for more advanced math and science courses, such as calculus. The topics you listed are all important concepts and techniques that students need to understand in order to be successful in calculus and other advanced math and science courses.

Rational exponents involve expressing numbers with fractional exponents, which is a way of extending the concept of powers to include non-integer exponents. Circles involve studying the properties of circles and their equations. Functions are mathematical objects that represent relationships between inputs and outputs, and understanding their properties is essential for studying calculus. Complex numbers are numbers that include both real and imaginary components and are used extensively in advanced math and science courses.

PREREQUISITES 

Synthetic division is a technique for dividing polynomials by linear factors. Inverse functions are functions that undo the effects of other functions, and they are important for understanding calculus. Exponential and logarithmic functions are functions that involve the constants e and log base 10 or log base e, respectively, and they are used extensively in science and engineering.

Overall, precalculus is an important course for students who are interested in pursuing advanced math and science courses. By mastering the concepts and techniques covered in this course, students will be well-prepared for the challenges of calculus and beyond.

МАТ143 Mathematical Analysis HELP（EXAM HELP， ONLINE TUTOR）

a. To show that $f(x,y) = \frac{1}{\sqrt{x^2 + y^2}}$ is Lebesgue-integrable on the square $[0,1] \times [0,1]$, we need to show that its integral over this square is finite. We can do this by calculating the integral:

$$\int_{0}^{1} \int_{0}^{1} \frac{1}{\sqrt{x^2+y^2}} dxdy$$

To evaluate this integral, we can use polar coordinates. Let $r = \sqrt{x^2 + y^2}$ and $\theta = \tan^{-1}(y/x)$. Then, we have:

$$\int_{0}^{1} \int_{0}^{1} \frac{1}{\sqrt{x^2+y^2}} dxdy = \int_{0}^{\pi/2} \int_{0}^{1} \frac{1}{r} r dr d\theta = \int_{0}^{\pi/2} 1 d\theta = \frac{\pi}{2}$$

Since the integral is finite, we can conclude that $f(x,y)$ is Lebesgue-integrable on the square $[0,1] \times [0,1]$.

b. To evaluate the integral $\int_{0}^{1} \int_{0}^{1} \frac{x-y}{(x+y)^3} dxdy$, we can try to use Fubini’s theorem to switch the order of integration. However, Fubini’s theorem does not apply because the integrand $\frac{x-y}{(x+y)^3}$ is not absolutely integrable on the square $[0,1] \times [0,1]$. To see why, consider the integral:

$$\int_{0}^{1} \int_{0}^{1} \left| \frac{x-y}{(x+y)^3} \right| dxdy$$

Using the inequality $|x-y| \leq \sqrt{2}(x^2+y^2)$, we can bound the integrand:

$$\left| \frac{x-y}{(x+y)^3} \right| \leq \frac{\sqrt{2}}{(x+y)^2}$$

Therefore, we have:

$$\int_{0}^{1} \int_{0}^{1} \left| \frac{x-y}{(x+y)^3} \right| dxdy \leq \sqrt{2} \int_{0}^{1} \int_{0}^{1} \frac{1}{(x+y)^2} dxdy$$

We can evaluate the inner integral using a similar polar coordinate transformation as before:

$$\int_{0}^{1} \frac{1}{(x+y)^2} dx = \int_{\tan^{-1}(y)}^{\pi/2} \frac{1}{\sec^2 \theta + y \tan \theta} d\theta$$

This integral diverges as $y \rightarrow 0$ because the integrand approaches a constant multiple of $\frac{1}{y}$ as $\theta \rightarrow \tan^{-1}(y)$.

Therefore, the integral $\int_{0}^{1} \int_{0}^{1} \frac{x-y}{(x+y)^3} dxdy$ does not exist in the usual sense. A similar argument shows that $\int_{0}^{1} \int_{0}^{1} \frac{x-y}{

a. To show that $\mathcal{B}_1 \times \mathcal{B}_1 = \mathcal{B}_2$, we need to show that every subset of $\mathbb{R}^2$ that is generated by the product of two open sets in $\mathbb{R}$ is a Borel set in $\mathbb{R}^2$, and vice versa.

Let $U,V \subseteq \mathbb{R}$ be open sets. Then, the product $U \times V = {(x,y) \in \mathbb{R}^2 : x \in U, y \in V}$ is an open set in $\mathbb{R}^2$ with respect to the product topology. Therefore, $U \times V$ is a Borel set in $\mathbb{R}^2, since the product topology is generated by the collection of all sets of the form $U \times V$ where $U,V \subseteq \mathbb{R}$ are open.

Conversely, let $B \subseteq \mathbb{R}^2$ be a Borel set in $\mathbb{R}^2$. Then, by definition, $B$ can be generated by the open sets in $\mathbb{R}^2$, which include sets of the form $U \times V$, where $U,V \subseteq \mathbb{R}$ are open. Therefore, $B$ can be expressed as a countable union and intersection of sets of the form $U \times V$, which implies that $B$ belongs to the Borel $\sigma$-algebra generated by the sets of the form $U \times V$. Hence, $B$ is a product of two Borel sets in $\mathbb{R}$, and hence $B$ belongs to $\mathcal{B}_1 \times \mathcal{B}_1$.

Therefore, we have shown that $\mathcal{B}_1 \times \mathcal{B}_1 = \mathcal{B}_2$.

b. To show that $\mathcal{L}_1 \times \mathcal{L}_1 \neq \mathcal{L}_2$, we will show that there exists a set $B \subseteq \mathbb{R}^2$ that belongs to $\mathcal{L}_2$ but not to $\mathcal{L}_1 \times \mathcal{L}_1$.

Consider the set $B = {(x,x) : x \in [0,1]}$. This set is the diagonal line segment in the unit square $[0,1] \times [0,1]$.

To show that $B$ belongs to $\mathcal{L}_2$, note that $B$ can be expressed as the inverse image of the function $f(x,y) = x – y$ evaluated at the value $0$. That is, $B = f^{-1}(0)$, where $f : \mathbb{R}^2 \to \mathbb{R}$ is a continuous function, and hence $B$ is a zero set, and thus belongs to $\mathcal{L}_2$.

On the other hand, $B$ does not belong to $\mathcal{L}_1 \times \mathcal{L}_1$ because $B$ cannot be expressed as a product of two Lebesgue measurable sets in $\mathbb{R}$.

Textbooks

• An Introduction to Stochastic Modeling, Fourth Edition by Pinsky and Karlin (freely
available through the university library here)
• Essentials of Stochastic Processes, Third Edition by Durrett (freely available through
the university library here)
To reiterate, the textbooks are freely available through the university library. Note that
you must be connected to the university Wi-Fi or VPN to access the ebooks from the library
links. Furthermore, the library links take some time to populate, so do not be alarmed if
the webpage looks bare for a few seconds.

Statistics-lab™可以为您提供ucc.edu МАТ143 Mathematical Analysis数学分析的代写代考和辅导服务！ 请认准Statistics-lab™. Statistics-lab™为您的留学生涯保驾护航。