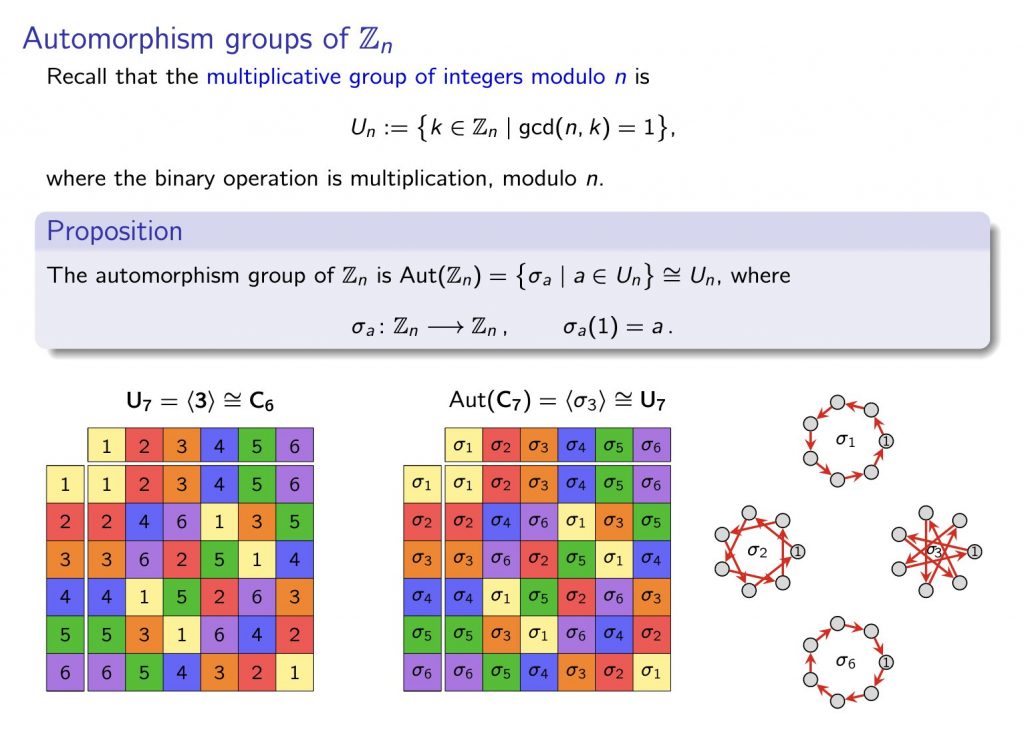
数学代写|现代代数代写Modern Algebra代考|MATH067
如果你也在 怎样代写现代代数Modern Algebra 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。现代代数Modern Algebra就像数学的其他分支一样——只有从最基本的思想和例子中仔细地推导才能掌握。但这需要时间,而且有些目标在你实现之前是不明确的。
现代代数Modern Algebra这门学科的思想和方法几乎渗透到现代数学的每一个部分。此外,没有一门学科更适合培养处理抽象概念的能力,即理解和处理问题或学科的基本要素。这包括阅读数学的能力,提出正确的问题,解决问题,运用演绎推理,以及写出正确、切中要害、清晰的数学。
statistics-lab™ 为您的留学生涯保驾护航 在代写现代代数Modern Algebra方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写现代代数Modern Algebra代写方面经验极为丰富,各种代写现代代数Modern Algebra相关的作业也就用不着说。
数学代写|现代代数代写Modern Algebra代考|The Field of Quotients of an Integral Domain
The example of an integral domain that is most familiar to us is the set $\mathbf{Z}$ of all integers, and the most familiar example of a field is the set of all rational numbers. There is a very natural and intimate relationship between these two systems. In fact, a rational number is by definition a quotient $a / b$ of integers $a$ and $b$, with $b \neq 0$; that is, the set of rational numbers is the set of all quotients of integers with nonzero denominators. For this reason, the set of rational numbers is frequently referred to as “the quotient field of the integers.” In this section, we shall see that an analogous field of quotients can be constructed for an arbitrary integral domain.
Before we present this construction, let us review the basic definitions of equality, addition, and multiplication in the rational numbers. We recall that for rational numbers $\frac{a}{b}$ and $\frac{c}{d}$,
$$
\begin{aligned}
& \frac{a}{b}=\frac{c}{d} \text { if and only if } a d=b c, \
& \frac{a}{b}+\frac{c}{d}=\frac{a d+b c}{b d}, \
& \frac{a}{b} \cdot \frac{c}{d}=\frac{a c}{b d} .
\end{aligned}
$$
Note that the definitions of equality, addition, and multiplication for rational numbers are based on the corresponding definitions for the integers. These definitions guide our construction of the quotient field for an arbitrary integral domain $D$.
Our first step in this construction is the following definition.
A Relation on Ordered Pairs
Let $D$ be an integral domain and let $S$ be the set of all ordered pairs $(a, b)$ of elements of $D$ with $b \neq 0$ :
$$
S={(a, b) \mid a, b \in D \text { and } b \neq 0}
$$
The relation $\sim$ is defined on $S$ by
$(a, b) \sim(c, d)$ if and only if $a d=b c$.
The relation $\sim$ is an obvious imitation of the equality of rational numbers, and we can show that it is indeed an equivalence relation on $S$.
数学代写|现代代数代写Modern Algebra代考|The Equivalence Relation
The relation $\sim$ in Definition 5.21 is an equivalence relation on $S$.
Proof We shall show that $\sim$ is reflexive, symmetric, and transitive. Let $(a, b),(c, d)$, and $(f, g)$ be arbitrary elements of $S$.
$(a, b) \sim(a, b)$, since the commutative multiplication in $D$ implies that $a b=b a$.
$(a, b) \sim(c, d) \Rightarrow a d=b c$
by definition of
$\Rightarrow d a=c b$ or $c b=d a$
since multiplication is commutative in $D$
$\Rightarrow(c, d) \sim(a, b)$
by definition of $\sim$.
Assume that $(a, b) \sim(c, d)$ and $(c, d) \sim(f, g)$.
$$
\left.\begin{array}{l}
(a, b) \sim(c, d) \Rightarrow a d=b c \Rightarrow a d g=b c g \
(c, d) \sim(f, g) \Rightarrow c g=d f \Rightarrow b c g=b d f
\end{array}\right} \Rightarrow a d g=b d f
$$
Using the commutative property of multiplication in $D$ once again, we have ${ }^{\dagger}$
$$
d a g=d b f
$$
where $d \neq 0$, and therefore
$$
a g=b f
$$
by Theorem 5.16. According to Definition 5.21, this implies that $(a, b) \sim(f, g)$.
Thus $\sim$ is an equivalence relation on $S$.
The next definition reveals the basic plan for our construction of the quotient field of $D$.
Let $D, S$, and $\sim$ be the same as in Definition 5.21 and Lemma 5.22. For each $(a, b)$ in $S$, let $[a, b]$ denote the equivalence class in $S$ that contains $(a, b)$, and let $Q$ denote the set of all equivalence classes $[a, b]$ :
$$
Q={[a, b] \mid(a, b) \in S} .
$$
The set $Q$ is called the set of quotients for $D$.
We shall at times need the fact that for any $x \neq 0$ in $D$ and any $[a, b]$ in $Q$,
$$
[a, b]=[a x, b x] .
$$
This follows at once from the equality $a(b x)=b(a x)$ in the integral domain $D$.
现代代数代考
数学代写|现代代数代写Modern Algebra代考|The Field of Quotients of an Integral Domain
我们最熟悉的一个积分定义域的例子是所有整数的集合$\mathbf{Z}$,最熟悉的一个域的例子是所有有理数的集合。这两个系统之间有着非常自然和密切的关系。事实上,有理数根据定义是整数$a$和$b$的商$a / b$,其中$b \neq 0$;也就是说,有理数的集合是所有分母为非零的整数的商的集合。由于这个原因,有理数的集合经常被称为“整数的商域”。在本节中,我们将看到,对于任意积分域,可以构造一个类似的商域。
在我们介绍这种构造之前,让我们回顾一下有理数中相等、加法和乘法的基本定义。回想一下,对于有理数$\frac{a}{b}$和$\frac{c}{d}$,
$$
\begin{aligned}
& \frac{a}{b}=\frac{c}{d} \text { if and only if } a d=b c, \
& \frac{a}{b}+\frac{c}{d}=\frac{a d+b c}{b d}, \
& \frac{a}{b} \cdot \frac{c}{d}=\frac{a c}{b d} .
\end{aligned}
$$
请注意,有理数的相等、加法和乘法的定义是基于整数的相应定义。这些定义指导我们构造任意积分域$D$的商域。
这个构造的第一步是下面的定义。
有序对上的关系
设$D$为整域,设$S$为$D$与$b \neq 0$的元素的所有有序对$(a, b)$的集合:
$$
S={(a, b) \mid a, b \in D \text { and } b \neq 0}
$$
关系$\sim$在$S$上由
$(a, b) \sim(c, d)$当且仅当$a d=b c$。
关系$\sim$是有理数等式的明显模仿,我们可以在$S$上证明它确实是一个等价关系。
数学代写|现代代数代写Modern Algebra代考|The Equivalence Relation
定义5.21中的关系$\sim$是$S$上的等价关系。
我们将证明$\sim$是自反的、对称的和可传递的。设$(a, b),(c, d)$和$(f, g)$为$S$的任意元素。
$(a, b) \sim(a, b)$,因为$D$中的交换乘法意味着$a b=b a$。
$(a, b) \sim(c, d) \Rightarrow a d=b c$
根据的定义
$\Rightarrow d a=c b$或$c b=d a$
因为乘法在$D$中是可交换的
$\Rightarrow(c, d) \sim(a, b)$
根据$\sim$的定义。
假设$(a, b) \sim(c, d)$和$(c, d) \sim(f, g)$。
$$
\left.\begin{array}{l}
(a, b) \sim(c, d) \Rightarrow a d=b c \Rightarrow a d g=b c g \
(c, d) \sim(f, g) \Rightarrow c g=d f \Rightarrow b c g=b d f
\end{array}\right} \Rightarrow a d g=b d f
$$
利用$D$的乘法交换性,再一次得到${ }^{\dagger}$
$$
d a g=d b f
$$
哪里$d \neq 0$,因此
$$
a g=b f
$$
根据定理5.16。根据定义5.21,这意味着$(a, b) \sim(f, g)$。
因此$\sim$是$S$上的等价关系。
下一个定义揭示了我们构造$D$的商域的基本计划。
设$D, S$和$\sim$与定义5.21和引理5.22中的相同。对于$S$中的每个$(a, b)$,让$[a, b]$表示$S$中包含$(a, b)$的等价类,让$Q$表示所有等价类的集合$[a, b]$:
$$
Q={[a, b] \mid(a, b) \in S} .
$$
集合$Q$称为$D$的商集。
我们有时需要这样一个事实:对于$D$中的任何$x \neq 0$和$Q$中的任何$[a, b]$,
$$
[a, b]=[a x, b x] .
$$
这是由积分域$D$的等式$a(b x)=b(a x)$得出的。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。