数学代写|微积分代写Calculus代写|MTH-211
如果你也在 怎样代写微积分Calculus 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。微积分Calculus 基本上就是非常高级的代数和几何。从某种意义上说,它甚至不是一门新学科——它采用代数和几何的普通规则,并对它们进行调整,以便它们可以用于更复杂的问题。(当然,问题在于,从另一种意义上说,这是一门新的、更困难的学科。)
微积分Calculus数学之所以有效,是因为曲线在局部是直的;换句话说,它们在微观层面上是直的。地球是圆的,但对我们来说,它看起来是平的,因为与地球的大小相比,我们在微观层面上。微积分之所以有用,是因为当你放大曲线,曲线变直时,你可以用正则代数和几何来处理它们。这种放大过程是通过极限数学来实现的。
statistics-lab™ 为您的留学生涯保驾护航 在代写微积分Calculus方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写微积分Calculus代写方面经验极为丰富,各种代写微积分Calculus相关的作业也就用不着说。
数学代写|微积分代写Calculus代写|ThE Growth OF KinEMatics IN thE WeST
Whereas in dynamics movement is studied in relation to the forces associated with it, in kinematics only its spatial and temporal aspects are considered. It follows that in kinematics we are concerned with the description of movement and not with its causes and it can therefore properly be regarded as the geometry of movement.
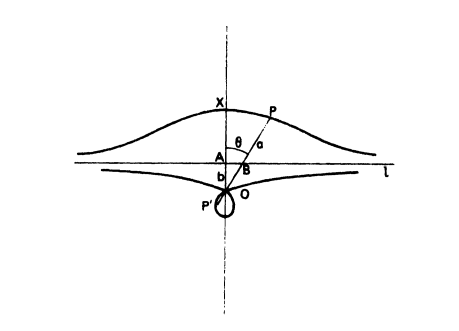
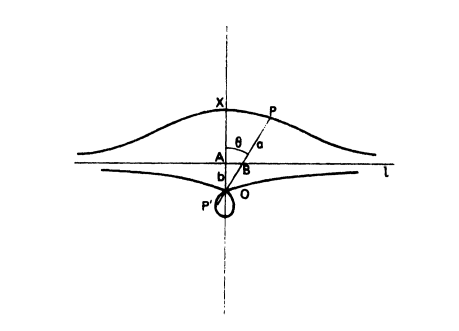
If a particle be known to trace a given curve the geometric properties of that curve can be used to predict the subsequent positions of the particle; conversely, if a curve be defined as the path of a point moving under specified conditions, then the laws of kinematics can be utilised to provide information as to certain geometric properties of the curve. For example, knowledge of the instantaneous motion at a given point on the curve enables us to draw the tangent to the curve at that point. The development, in the fourteenth century, of certain important concepts of motion including instantaneous velocity can therefore be seen to have direct and immediate bearing on the study of the tangent properties of curves. Furthermore, the introduction of graphical methods of representation led to the establishment of a link between the velocity-time graph, the total distance covered and the area under the curve, and this in turn is closely connected with the integral calculus (see Figs. 2.8 and 2.9).
The imaginative insights gained by the use of kinematic concepts in geometry were responsible for some of the more powerful methods developed during the seventeenth century for the study of curves. The work of Isaac Barrow, for instance, which certainly influenced Newton, is dominated by the idea of curves generated by moving points and lines.
数学代写|微积分代写Calculus代写|The Latitude OF Forms
At Merton College, Oxford, between the years 1328 and 1350, the distinction between kinematics and dynamics was made explicit. In the work of Thomas Bradwardine, William Heytesbury, Richard Swineshead and John Dumbleton the foundations for further study in this field were laid through the clarification and formalisation of a number of important concepts including the notion of instantaneous velocity (velocitas instantanea). ${ }^{\dagger}$
The study of space and motion at Merton College arose from the mediaeval discussion of the intension and remission of forms, i.e. the increase and decrease of the intensity of qualities. The distinction between intension and extension is exemplified in the case of heat by the difference between temperature, or degree of heat, and quantity of heat; in the case of weight between density, or weight per unit volume and total weight. For local motion the distinction is between velocity (or motion) at a given instant (instantaneous velocity) and total motion over a period of time, i.e. distance covered.
William Heytesbury distinguishes between uniform and difform (nonuniform) motion. In the case of difform motion he says: $\ddagger if, in a period of time, it were moved uniformly at the same degree of velocity (uniformiter illo gradu velocitatis) with which it is moved in that given instant, whatever [instant] be assigned.
The most notable single achievement of the Merton School was the establishment of the mean speed theorem for uniformly accelerated (uniformly difform) motion, i.e. $s=\left(v_1+v_2\right) t / 2$, where $s$ is the distance covered in time $t$ and $v_1$ and $v_2$ are the initial and final velocities. Various proofs of this theorem were presented, some purely arithmetical and others depending on some skilful manipulation of infinite series. Although in some cases velocities (or intensions) were represented by single straight lines and Richard Swineshead actually uses a geometric analogy ${ }^{\dagger}$ to explain intension and remission of qualities it was not until knowledge of the Merton College work reached France and Italy that it received the full benefits of geometric representation and so linked kinematics with the geometry of straight and curved lines.

微积分代考
数学代写|微积分代写Calculus代写|ThE Growth OF KinEMatics IN thE WeST
在动力学中,运动是根据与之相关的力来研究的,而在运动学中,只考虑其空间和时间方面。由此可见,在运动学中,我们所关心的是运动的描述,而不是运动的原因,因此,运动学可以恰当地看作是运动的几何学。
如果已知粒子沿给定曲线运动,则该曲线的几何特性可用于预测粒子的后续位置;相反,如果一条曲线被定义为一个点在特定条件下运动的路径,那么运动学定律可以用来提供关于曲线的某些几何性质的信息。例如,知道曲线上某一点的瞬时运动,我们就能画出该点与曲线的切线。因此,在14世纪,包括瞬时速度在内的某些重要运动概念的发展,可以看作对曲线的切线性质的研究有直接和直接的影响。此外,图形表示方法的引入使速度-时间图、覆盖的总距离和曲线下的面积之间建立了联系,而这又与积分学密切相关(见图2.8和图2.9)。
利用几何学中的运动学概念所获得的富有想象力的洞见,促成了17世纪研究曲线的一些更有力的方法的发展。例如,艾萨克·巴罗(Isaac Barrow)的工作,当然影响了牛顿,主要是由移动的点和线产生曲线的想法。
数学代写|微积分代写Calculus代写|The Latitude OF Forms
1328年至1350年间,牛津大学默顿学院明确区分了运动学和动力学。在Thomas Bradwardine, William Heytesbury, Richard Swineshead和John Dumbleton的工作中,通过澄清和形式化一些重要的概念,包括瞬时速度(velocitas instantanea)的概念,为该领域的进一步研究奠定了基础。${} ^{\匕首}$
默顿学院对空间和运动的研究源于中世纪对形式的强度和缓解的讨论,即质量强度的增加和减少。在热的情况下,强度和延伸之间的区别可以通过温度或热度与热量之间的差异来举例说明;在密度或单位体积重量与总重量之间的情况下。对于局部运动,区别在于给定瞬间(瞬时速度)的速度(或运动)和一段时间内的总运动,即所走过的距离。
威廉·海茨伯里区分了均匀运动和非均匀运动。在不均匀运动的情况下,他说:“如果在一段时间内,物体以与它在给定瞬间的运动速度相同的速度均匀运动,无论给定的是什么。”
默顿学派最著名的成就是建立了均匀加速(均匀均匀)运动的平均速度定理,即$s=\左(v_1+v_2\右)t / 2$,其中$s$是时间所覆盖的距离$t$, $v_1$和$v_2$是初始和最终速度。给出了这个定理的各种证明,有些是纯粹的算术证明,有些则依赖于对无穷级数的一些巧妙的处理。虽然在某些情况下,速度(或强度)是用一条直线来表示的,Richard Swineshead实际上使用了一个几何类比来解释强度和质量的缓解,但直到默顿学院的研究成果传播到法国和意大利,它才充分受益于几何表示,并将运动学与直线和曲线的几何联系起来。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。