数学代写|微积分代写Calculus代写|MTH-211
如果你也在 怎样代写微积分Calculus 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。微积分Calculus 基本上就是非常高级的代数和几何。从某种意义上说,它甚至不是一门新学科——它采用代数和几何的普通规则,并对它们进行调整,以便它们可以用于更复杂的问题。(当然,问题在于,从另一种意义上说,这是一门新的、更困难的学科。)
微积分Calculus数学之所以有效,是因为曲线在局部是直的;换句话说,它们在微观层面上是直的。地球是圆的,但对我们来说,它看起来是平的,因为与地球的大小相比,我们在微观层面上。微积分之所以有用,是因为当你放大曲线,曲线变直时,你可以用正则代数和几何来处理它们。这种放大过程是通过极限数学来实现的。
statistics-lab™ 为您的留学生涯保驾护航 在代写微积分Calculus方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写微积分Calculus代写方面经验极为丰富,各种代写微积分Calculus相关的作业也就用不着说。
数学代写|微积分代写Calculus代写|JOHANN KEPLBR
Among the first of the seventeenth-century mathematicians to abandon the formal proof structure of Archimedes and to introduce the free use of infinitesimals in the determination of areas and volumes was Johann Kepler. As an astronomer Kepler was primarily concerned to interpret the universe in terms of ordered mathematical harmony and throughout his entire life he pursued this goal with tireless energy and unremitting determination. In so doing he was willing to go to any lengths to develop from a mathematical hypothesis sufficient data to test a theory but he was nevertheless prepared at any time to abandon the theory and start afresh should it fail to fit the observed facts. ${ }^{\dagger}$
As a mathematician Kepler sought to demonstrate in the simplest possible way the existence of mathematical form and structure in the external world and, upheld by his Platonic-Pythagorean philosophy, he often allowed faith, analogy and intuition to guide him when traditional methods failed. Many of the mathematical problems which Kepler attempted to resolve in the interests of astronomy were extremely difficult by any standards and well beyond the scope of existing seventeenth-century techniques. Although Kepler became conversant with the whole range of Greek mathematics he was more interested in results than in proofs. If an Archimedean demonstration supplied him with a required property he was glad to make use of it, but when formal methods failed to provide the answers to his problems the practical necessities of the situation drove him onwards to devise new techniques and processes.
Many of the astronomical problems with which he was faced required the evaluation of trigonometric integrals and in some of these cases, finding no theoretical method available, Kepler was obliged to resort to numerical summation. One such problem arose in connection with the theory of planetary magnetism $^{\dagger}$ in which the entire sphere of the planet was regarded as a magnet, one pole being attracted and the other repelled by the sun. After many attempts to determine the relation between the attractive and repulsive forces he finally concluded that it was necessary to divide off the arc into infinitely many sman and equal parts and to find the sum of the sines (or chords) of all the arcs. This was duly accomplished and he arrived (presumably by incomplete induction) at a conclusion formally equivalent to the definite integral
$$
\int_0^\alpha \sin \theta d \theta=1-\cos \alpha .
$$
数学代写|微积分代写Calculus代写|INDIVISIBLES IN ITALY
Although there is no record of any direct exchange of views between Kepler and Galileo on the subject of infinitesimals the publication of the Stereometria may well have acted as a spur, both to Galileo and to his pupil Cavalieri, in extending and developing their own work in this field. Although Galileo never actually published a work on indivisible methods as such the whole trend of the discussion in the Two New Sciences” throws light not only on his own approach to such matters but also on the background of ideas which he transmitted to Cavalieri.
Galileo had a profound respect for the Greeks and in consequence greatly admired the achievements of those mathematicians such as Valerio who had expanded and developed the work of Archimedes.” Nevertheless it is clear that for the most part his own ideas led him along entirely different paths. The influence of Scholastic thought on Galileo is, of course, most pronounced in his early works but even in Two New Sciences it is very much to the fore when he discusses the nature of indivisibles and the infinite. In this work Galileo reviews most of the mediaeval paradoxes and at the same time provides some new ones of his own. Most of these paradoxes are based on the concept of indivisible quantities and Galileo makes no distinction between physical atoms and line and surface elements: in fact he finds support for his belief in the efficacy of mathematical indivisibles from his desire to demonstrate the possibility of the existence of a vacuum.
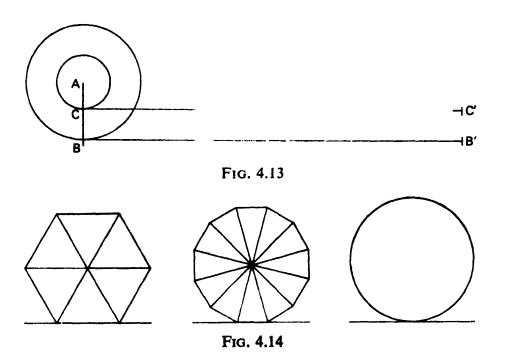
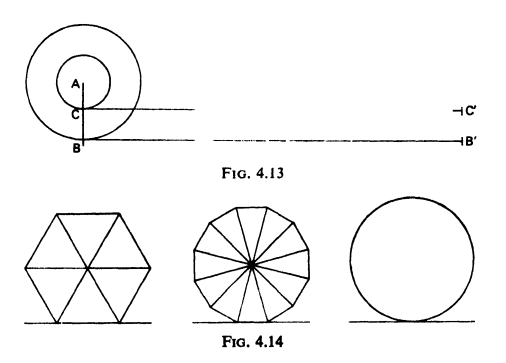
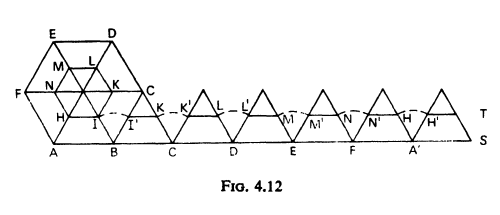
In discussing the Wheel of Aristotle, $\ddagger$ still a popular subject in the seventeenth century, Galileo first allows the hexagon, $A B C D E F$, to roll upon the line $A S$ (see Fig. 4.12) carrying with it a concentric hexagon, $H I K L M N$, inscribed within it. As the sides $A B, B C, C D, D E, E F, F A$ take up successive positions on the line $A B$, the sides of the concentric hexagon fall into positions $H I, I^{\prime} K, K^{\prime} L, \ldots, N^{\prime} H$ on the line $H T$, parallel to $A S$. Hence, in one revolution of the wheel, the total distance $H H^{\prime}$ moved by the point $H$ along the line $H T$ equals the distance $A A^{\prime}$ moved by the point $A$ along the line $A S$. The distance $H H^{\prime}$, however, is greater than the perimeter of the lesser polygon by the length of the spaces $I I^{\prime}, K K^{\prime}, L L^{\prime}, \ldots, H H^{\prime}$. Now, if the number of sides of the polygon be increased to 1000 , then in one complete revolution the lesser polygon will lay off 1000 sides and the same number of spaces along the line $H T$. Finally, if the rolling polygons be replaced by concentric circles, rigidly connected, the point $C$ on the lesser circle will move through the same distance, $C C^{\prime}$, as the point $B$ on the greater circle (see Fig. 4.13).
微积分代考
数学代写|微积分代写Calculus代写|JOHANN KEPLBR
约翰·开普勒是17世纪最早抛弃阿基米德的形式证明结构,并在确定面积和体积时引入无限小的自由使用的数学家之一。作为一名天文学家,开普勒主要关注的是用有序的数学和谐来解释宇宙,在他的一生中,他以不懈的精力和不懈的决心追求这一目标。在这样做的时候,他愿意尽一切努力从数学假设中发展出足够的数据来检验一种理论,但他也随时准备放弃这种理论,如果它与观察到的事实不相符,他又重新开始。 ${ }^{\dagger}$
作为一名数学家,开普勒试图以最简单的方式证明数学形式和结构在外部世界的存在。在他的柏拉图-毕达哥拉斯哲学的支持下,当传统方法失败时,他经常让信仰、类比和直觉来指导他。开普勒为了天文学的兴趣而试图解决的许多数学问题,以任何标准来看都是极其困难的,而且远远超出了17世纪现有技术的范围。尽管开普勒对整个希腊数学都很熟悉,但他对结果比对证明更感兴趣。如果阿基米德的论证为他提供了所需的性质,他很乐意利用它,但是当正式的方法不能为他的问题提供答案时,实际情况的需要驱使他继续设计新的技术和过程。
他所面临的许多天文问题都需要计算三角积分,在某些情况下,由于找不到可用的理论方法,开普勒不得不求助于数值求和。其中一个这样的问题出现在行星磁学理论$^{\dagger}$中,在这个理论中,行星的整个球体被视为一个磁体,一极被太阳吸引,另一极被太阳排斥。在多次尝试确定引力和斥力之间的关系之后,他最终得出结论,有必要将弧线分成无限多等分,并找出所有弧线的正弦(或弦)之和。这是如期完成的,他(大概是通过不完全归纳法)得出了一个形式上等同于定积分的结论
$$
\int_0^\alpha \sin \theta d \theta=1-\cos \alpha .
$$
数学代写|微积分代写Calculus代写|INDIVISIBLES IN ITALY
尽管没有记录表明开普勒和伽利略在无穷小问题上有过任何直接的意见交换,立体测量的出版对伽利略和他的学生卡瓦列里来说都是一种刺激,在这一领域扩展和发展他们自己的工作。虽然伽利略从未真正发表过关于不可分割方法的著作,但《两门新科学》中讨论的整个趋势不仅揭示了他自己对这些问题的研究方法,也揭示了他传递给卡瓦列里的思想背景。
伽利略对希腊人有很深的敬意,因此非常钦佩那些数学家的成就,比如瓦莱里奥,他们扩展和发展了阿基米德的工作。”然而,很明显,在很大程度上,他自己的思想引导他走上了完全不同的道路。经院思想对伽利略的影响,当然,在他早期的作品中是最明显的,但即使在《两门新科学》中,当他讨论不可分割和无限的本质时,这种影响也非常突出。在这部著作中,伽利略回顾了大部分中世纪的悖论,同时也提出了一些他自己的新悖论。这些悖论大多是基于不可分量的概念,伽利略对物理原子、线元素和面元素没有区别:事实上,他从证明真空存在的可能性的愿望中找到了对数学不可分有效性的信念的支持。
在讨论亚里士多德的轮子时(在17世纪仍然是一个流行的话题),伽利略首先允许六边形(a B C D E F)在线上滚动(见图4.12),它带着一个同心六边形(H I K L M N),上面刻着一个同心六边形(H I K L M N)。由于边$A B, B C, C D, D E, E F, F A$在直线$A B$上占据连续位置,同心六边形的边落在直线$H T$上的位置$H I, $ I^{\素数}K, K^{\素数}L, $ ldots, N^{\素数}H$,平行于$A S$。因此,在车轮转一圈时,H H^{\ ‘}$被点H$沿直线H T$移动的总距离等于A A^{\ ‘}$被点A$沿直线A S$移动的距离。然而,距离$H H^{\prime}$大于较小多边形的周长$I I^{\prime}, K K^{\prime}, L L^{\prime}, \ldots, H H^{\prime}$。现在,如果多边形的边数增加到1000条,那么在一次完整的旋转中,较小的多边形将沿直线H T$释放1000条边和相同数量的空间。最后,如果将滚动多边形替换为刚性连接的同心圆,则较小圆上的点$C$将与较大圆上的点$B$移动相同的距离$C C^{\ ‘}$(见图4.13)。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。