如果你也在 怎样代写微积分Calculus 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。微积分Calculus 最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
微积分Calculus 它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互关联,它们利用了无限序列和无限数列收敛到一个明确定义的极限的基本概念 。17世纪末,牛顿(Isaac Newton)和莱布尼兹(Gottfried Wilhelm Leibniz)独立开发了无限小数微积分。后来的工作,包括对极限概念的编纂,将这些发展置于更坚实的概念基础上。今天,微积分在科学、工程和社会科学中得到了广泛的应用。
statistics-lab™ 为您的留学生涯保驾护航 在代写微积分Calculus方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写微积分Calculus代写方面经验极为丰富,各种代写微积分Calculus相关的作业也就用不着说。
数学代写|微积分代写Calculus代写|Radioactivity
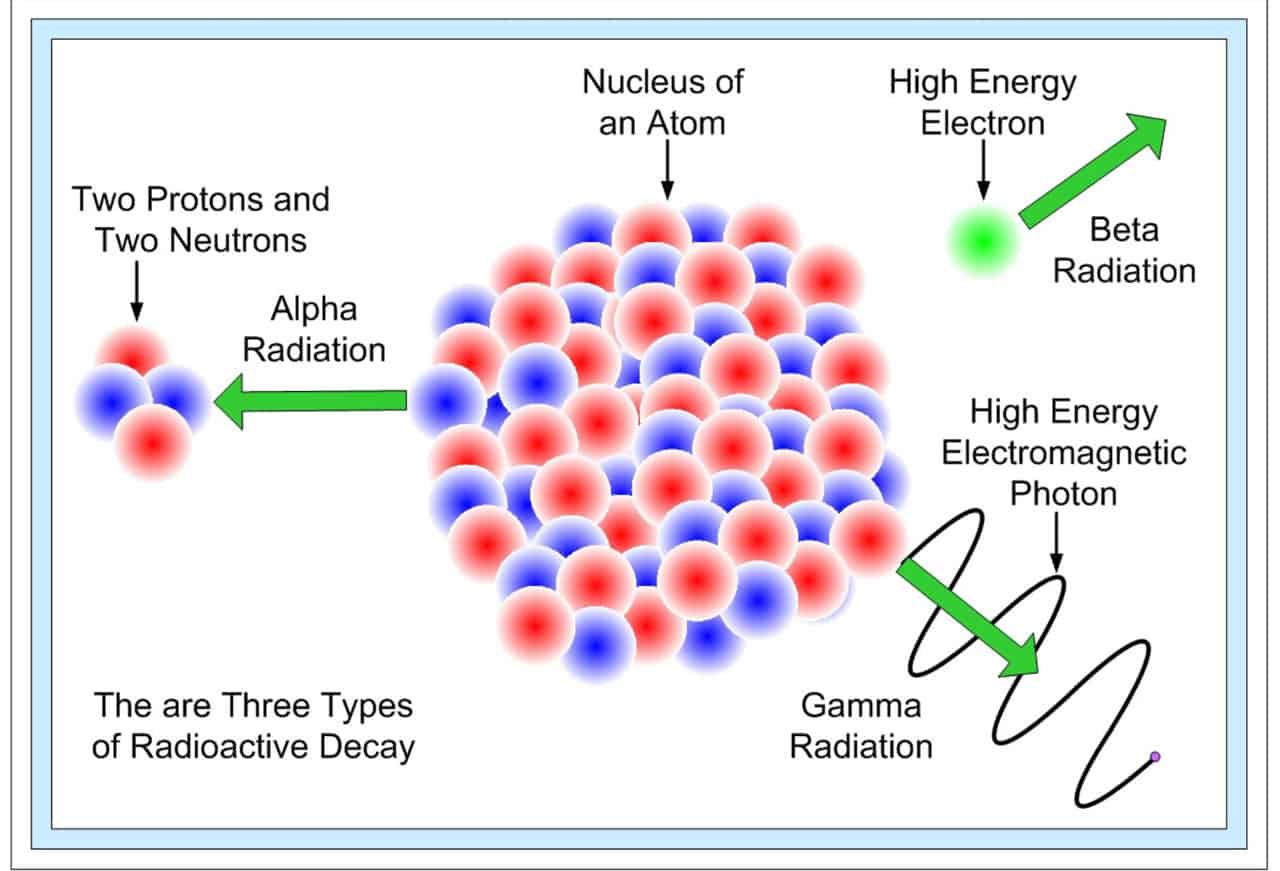
Some atoms are unstable and can spontaneously emit mass or radiation. This process is called radioactive decay, and an element whose atoms go spontaneously through this process is called radioactive. Sometimes when an atom emits some of its mass through this process of radioactivity, the remainder of the atom re-forms to make an atom of some new element. For example, radioactive carbon-14 decays into nitrogen; radium, through a number of intermediate radioactive steps, decays into lead.
Experiments have shown that at any given time the rate at which a radioactive element decays (as measured by the number of nuclei that change per unit time) is approximately proportional to the number of radioactive nuclei present. Thus, the decay of a radioactive element is described by the equation $d y / d t=-k y, k>0$. It is conventional to use $-k$, with $k>0$, to emphasize that $y$ is decreasing. If $y_0$ is the number of radioactive nuclei present at time zero, the number still present at any later time $t$ will be
$$
y=y_0 e^{-k t}, \quad k>0 .
$$
The half-life of a radioactive element is the time expected to pass until half of the radioactive nuclei present in a sample decay. It is an interesting fact that the half-life is a constant that does not depend on the number of radioactive nuclei initially present in the sample, but only on the radioactive substance.
To compute the half-life, let $y_0$ be the number of radioactive nuclei initially present in the sample. Then the number $y$ present at any later time $t$ will be $y=y_0 e^{-k t}$. We seek the value of $t$ at which the number of radioactive nuclei present equals half the original number:
$$
\begin{aligned}
y_0 e^{-k t} & =\frac{1}{2} y_0 \
e^{-k t} & =\frac{1}{2} \
-k t & =\ln \frac{1}{2}=-\ln 2 \quad \text { Reciprocal Rule for logarithms } \
t & =\frac{\ln 2}{k} .
\end{aligned}
$$
数学代写|微积分代写Calculus代写|Heat Transfer: Newton’s Law of Cooling
Hot soup left in a tin cup cools to the temperature of the surrounding air. A hot silver bar immersed in a large tub of water cools to the temperature of the surrounding water. In situations like these, the rate at which an object’s temperature is changing at any given time is roughly proportional to the difference between its temperature and the temperature of the surrounding medium. This observation is called Newton’s Law of Cooling, although it applies to warming as well.
If $H$ is the temperature of the object at time $t$ and $H_S$ is the constant surrounding temperature, then the differential equation is
$$
\frac{d H}{d t}=-k\left(H-H_S\right)
$$
If we substitute $y$ for $\left(H-H_S\right)$, then
$$
\begin{array}{rlrl}
\frac{d y}{d t} & =\frac{d}{d t}\left(H-H_S\right)=\frac{d H}{d t}-\frac{d}{d t}\left(H_S\right) & \
& =\frac{d H}{d t}-0 & & \
& =\frac{d H}{d t} & & H_S \text { is a constant. } \
& =-k\left(H-H_S\right) & \
& =-k y . & & \text { Eq. (8) } \
& & H-H_S=y
\end{array}
$$
We know that the solution of the equation $d y / d t=-k y$ is $y=y_0 e^{-k t}$, where $y(0)=y_0$. Substituting $\left(H-H_S\right)$ for $y$, this says that
$$
H-H_S=\left(H_0-H_S\right) e^{-k t},
$$
where $H_0$ is the temperature at $t=0$. This equation is the solution to Newton’s Law of Cooling.
微积分代考
数学代写|微积分代写Calculus代写|Radioactivity
有些原子是不稳定的,可以自发地释放质量或辐射。这个过程被称为放射性衰变,原子自发地经历这个过程的元素被称为放射性元素。有时,当一个原子通过这种放射性过程释放出它的一部分质量时,原子的其余部分就会重新形成某种新元素的原子。例如,放射性的碳-14会衰变成氮;镭经过若干中间放射性步骤,衰变成铅。
实验表明,在任何给定时间,放射性元素的衰变速率(以单位时间内变化的原子核数来衡量)与存在的放射性原子核数大致成正比。因此,放射性元素的衰变可以用公式$d y / d t=-k y, k>0$来描述。通常使用$-k$和$k>0$来强调$y$正在减少。如果$y_0$是在时间0存在的放射性核的数量,那么在以后任何时间仍然存在的数量$t$将是
$$
y=y_0 e^{-k t}, \quad k>0 .
$$
放射性元素的半衰期是指样品中存在的放射性原子核的一半衰变之前预期经过的时间。有趣的是,半衰期是一个常数,它不取决于样品中最初存在的放射性原子核的数目,而只取决于放射性物质。
为了计算半衰期,设$y_0$为样品中最初存在的放射性原子核的数目。那么在以后的任何时间出现的数字$y$$t$将是$y=y_0 e^{-k t}$。我们寻求$t$的值,在此值处存在的放射性核的数目等于原来数目的一半:
$$
\begin{aligned}
y_0 e^{-k t} & =\frac{1}{2} y_0 \
e^{-k t} & =\frac{1}{2} \
-k t & =\ln \frac{1}{2}=-\ln 2 \quad \text { Reciprocal Rule for logarithms } \
t & =\frac{\ln 2}{k} .
\end{aligned}
$$
数学代写|微积分代写Calculus代写|Heat Transfer: Newton’s Law of Cooling
放在锡杯里的热汤冷却到周围空气的温度。将一根热银棒浸入一大盆水中,冷却到与周围水的温度相同。在这种情况下,物体在任何给定时间的温度变化率大致与它的温度和周围介质的温度之差成正比。这一观察结果被称为牛顿冷却定律,尽管它也适用于变暖。
如果$H$是时刻$t$时物体的温度,$H_S$是恒定的周围温度,则微分方程为
$$
\frac{d H}{d t}=-k\left(H-H_S\right)
$$
如果我们用$y$代替$\left(H-H_S\right)$,那么
$$
\begin{array}{rlrl}
\frac{d y}{d t} & =\frac{d}{d t}\left(H-H_S\right)=\frac{d H}{d t}-\frac{d}{d t}\left(H_S\right) & \
& =\frac{d H}{d t}-0 & & \
& =\frac{d H}{d t} & & H_S \text { is a constant. } \
& =-k\left(H-H_S\right) & \
& =-k y . & & \text { Eq. (8) } \
& & H-H_S=y
\end{array}
$$
我们知道方程$d y / d t=-k y$的解是$y=y_0 e^{-k t}$,其中$y(0)=y_0$。将$\left(H-H_S\right)$代入$y$,得到
$$
H-H_S=\left(H_0-H_S\right) e^{-k t},
$$
$H_0$是$t=0$的温度。这个方程是牛顿冷却定律的解。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。