如果你也在 怎样代写微积分Calculus 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。微积分Calculus 基本上就是非常高级的代数和几何。从某种意义上说,它甚至不是一门新学科——它采用代数和几何的普通规则,并对它们进行调整,以便它们可以用于更复杂的问题。(当然,问题在于,从另一种意义上说,这是一门新的、更困难的学科。)
微积分Calculus数学之所以有效,是因为曲线在局部是直的;换句话说,它们在微观层面上是直的。地球是圆的,但对我们来说,它看起来是平的,因为与地球的大小相比,我们在微观层面上。微积分之所以有用,是因为当你放大曲线,曲线变直时,你可以用正则代数和几何来处理它们。这种放大过程是通过极限数学来实现的。
statistics-lab™ 为您的留学生涯保驾护航 在代写微积分Calculus方面已经树立了自己的口碑, 保证靠谱, 高质且原创的统计Statistics代写服务。我们的专家在代写微积分Calculus代写方面经验极为丰富,各种代写微积分Calculus相关的作业也就用不着说。

数学代写|微积分代写Calculus代写|Early Greex Mathematics and the Physical World
Early Egyptian mathematics consisted for the most part of empirical rules for performing operations on concrete objects by means of numerical processes, and generalisations which emerged seem to have resulted from the simple extension of a rule found to be valid in a number of special cases to all others. Babylonian texts, on the other hand, exhibit a much greater degree of generalisation but unfortunately we know little of the manner in which these generalisations were arrived at. With the Greeks, however, we find for the first time the idea of a general proof, valid in all cases. The developing concept of mathematics as a deductive system linked, at least in its initial assumptions and in its final conclusions with the physical world, led to increasing speculation as to the relation between the abstract elements of such a system and the concrete objects, shapes and forms belonging to the externai world. Much thought was given as to the fundamental basis of knowledge and to the processes of abstraction, idealisation, generalisation and deduction through which, in one way or another, such knowledge appeared to be derived.
The only physical science with any substantial basis of observation at this time was astronomy and, since in this case the objects observed were remote and their constitution unknown, it was natural that abstract and concrete elements should be regarded as interchangeable. ${ }^{\dagger}$
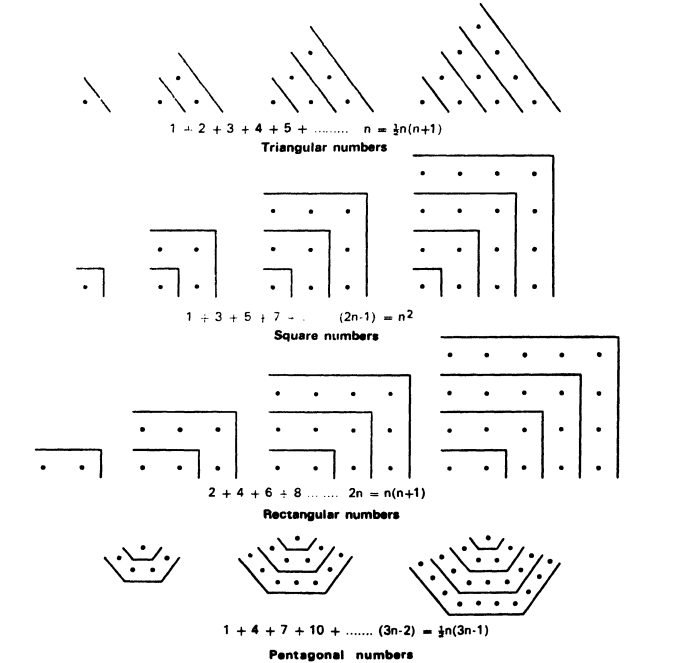
By a process of abstraction the early Pythagoreans developed the fruitfulconcept of figurate numbers, i.e. numbers made up of discrete elements arranged in geometric forms. $\ddagger$ This concept was then projected back upon the physical universe and its construction explained in terms of number. The discovery of the relation between musical harmony and the theory of proportion, attributed to Pythagoras himself, reinforced this view of the structure of the universe. The physical line segments and triangles of Pythagorean geometry could similarly be considered to be made up of discrete numerical elements until it was discovered that the diagonal of the square was incommensurable with the side. In this case, if the side be constructed of a finite number of discrete elements, how can the hypotenuse be constructed $?^{\dagger}$
Through geometry the idea of finite indivisible elements found a link with the materialist doctrine of physical atomism $\ddagger$ which arose at Abdera (ca. 430 B.C.). According to the Atomists, mind and soul as well as physical objects were considered to be made up of indivisible particles moving in the void. The belief that Democritus of Abdera also made use of the concept of indivisibles in volumetric determinations is confirmed by certain comments made by Archimedes in his treatise The Method. In particular, he attributes to Democritus, some fifty years before the formal proofs of Eudoxus, the discovery of the relationships between the volumes of a cone and a pyramid and those of a cylinder and a prism respectively.’ Of interest in this context is Plutarch’s account” of the queries said to have been raised by Democritus in connection with the sections of a cone cut by a plane parallel to the base.
数学代写|微积分代写Calculus代写|The Axiomatisation of Greek Mathematics
The final axiomatic structure of Greek mathematics was laid down in the third century B.C. and has been available for detailed scrutiny for over 2000 years in the Elements of Euclid, the treatises of Archimedes and the Conics of Apollonius.
It is never easy to establish with any degree of certitude the relationship between logic and philosophy on the one hand, and the structure of mathematical and scientific systems on the other. For this reason alone any attempt to assess the influence of the philosophy of Plato and the logic of Aristotle on the work of systematisation undertaken by Euclid and culminating in the thirteen books of the Elements is fraught with difficulty. Between the logic of Aristotle and the geometry of Euclid, at any rate, the interaction seems to have been mutual, for the impact of mathematics on Aristotle’s thought is only paralleled by the respect for formal logic displayed by Euclid in the Elements. This monumental work rapidly established itself as one of the supreme models of rigorous reasoning and has influenced all views on the nature of mathematical form and structure to the present day. The axiomatic or postulational approach laid down by Euclid was extended and developed in the field of geometry by Archimedes who not only showed himself a supreme master of the Euclidean method but made substantial and original advances by establishing the study of the physical sciences of statics and hydrostatics on a similar axiomatic basis.
In the Elements $\ddagger$ Euclid unified a collection of isolated discoveries and theorems into a single deductive system based upon a set of initial postulates, definitions and axioms. The definitions, although in some respects obscure and unhelpful, nevertheless suffice to identify the points, lines, planes and circles of physico-geometric experience and the postulates describe the technical requirements for their construction: the axioms (or common notions) represent rules of logic by means of which consequences may be deduced from the postulates. Although both Euclid and Archimedes make use of a great many logical processes not listed among Euclid’s axioms, those which are given constitute a satisfactory definition of equivalence of measure, a notion of fundamental significance in the construction of any formal deductive system.

微积分代考
数学代写|微积分代写Calculus代写|Early Greex Mathematics and the Physical World
早期的埃及数学大部分由经验法则组成,这些法则是通过数值过程对具体物体进行运算的,而普遍化似乎是由在一些特殊情况下有效的规则的简单推广而产生的。另一方面,巴比伦文本表现出更大程度的概括,但不幸的是,我们对这些概括的方式知之甚少。然而,在希腊人那里,我们第一次发现一般证明的观念,它在一切情况下都是有效的。数学作为一个演绎系统,至少在其最初的假设和最终的结论中,与物理世界联系在一起,这一概念的发展,导致人们越来越多地思考这种系统的抽象元素与属于外部世界的具体对象、形状和形式之间的关系。对于知识的基本基础,对于抽象、理想化、概括、演绎等过程,人们都作了大量的思考。
当时唯一有观测基础的自然科学是天文学,由于观测到的物体很遥远,它们的构成也不为人所知,所以很自然地,抽象元素和具体元素应该被认为是可以互换的。${} ^{\匕首}$
通过一个抽象的过程,早期的毕达哥拉斯学派发展了富有成果的形数概念,即由以几何形式排列的离散元素组成的数。这个概念随后被投射到物质宇宙上,它的结构用数字来解释。毕达哥拉斯发现了音乐和谐与比例理论之间的关系,这一发现强化了他对宇宙结构的看法。毕达哥拉斯几何中的物理线段和三角形同样可以被认为是由离散的数值元素组成的,直到人们发现正方形的对角线与边是不可通约的。在这种情况下,如果边是由有限个离散单元构成的,那么斜边是如何构成的呢?^{\匕首}$
通过几何学,有限的不可分割的元素的观念与在Abdera(约公元前430年)产生的物理原子论的唯物主义学说联系在一起。根据原子论者的观点,思想和灵魂以及物质对象都被认为是由在虚空中运动的不可分割的粒子组成的。阿基米德在他的论文《方法》中所作的某些评论证实了阿比德拉的德谟克利特在体积测定中也使用了不可分的概念。他特别指出,在欧多克索斯正式证明之前大约50年,德谟克利特发现了锥体和金字塔的体积与圆柱体和棱镜的体积之间的关系。在这种背景下,普鲁塔克对德谟克利特提出的问题的描述令人感兴趣,这些问题与平行于底座的平面切割的锥体部分有关。
数学代写|微积分代写Calculus代写|The Axiomatisation of Greek Mathematics
希腊数学的最终公理结构是在公元前3世纪确立的,并在2000多年前的欧几里得的《数学原理》、阿基米德的论文和阿波罗尼乌斯的《圆锥论》中得到了详细的研究。
一方面是逻辑和哲学,另一方面是数学和科学体系的结构,要确定两者之间的关系,从来都不是一件容易的事。仅仅因为这个原因,任何评估柏拉图的哲学和亚里士多德的逻辑对欧几里得的系统化工作的影响的尝试都充满了困难,欧几里得的系统化工作最终以13本《自然要素》告终。在亚里士多德的逻辑学和欧几里得的几何学之间,无论如何,似乎是相互作用的,因为数学对亚里士多德思想的影响,只有欧几里得在《几何原理》中对形式逻辑的尊重才能与之相提并论。这部巨著迅速确立了它作为严格推理的最高模型之一的地位,并影响了所有关于数学形式和结构本质的观点,直到今天。欧几里得提出的公理化或公设方法在几何领域得到了阿基米德的扩展和发展,他不仅表明自己是欧几里得方法的最高大师,而且通过在类似的公理化基础上建立静力学和流体静力学的物理科学研究,取得了实质性的原创进展。
在《数学基本原理》中,欧几里得将一系列孤立的发现和定理统一成一个基于一组初始公设、定义和公理的单一演绎系统。这些定义,虽然在某些方面模糊不清,毫无帮助,但足以识别物理几何经验的点、线、面和圆,以及公设描述了它们的构造的技术要求:公理(或共同概念)代表了逻辑规则,通过这些规则可以从公设中推导出结果。虽然欧几里得和阿基米德都使用了许多欧几里得公理中没有列出的逻辑过程,但这些过程构成了一个令人满意的度量等价的定义,这是一个在任何形式演绎系统的构造中具有根本意义的概念。
统计代写请认准statistics-lab™. statistics-lab™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。